Характерной
особенностью циклических кодов является
способность к распознаванию пакетов
ошибок. Под пакетом ошибок понимается
группирование ошибок в одной ограниченной
области кодового слова (рис. 3.16). Пакет
ошибок можно описать многочленом вида
.
(3.75)
Е
сли
длина пакета ошибок не превосходит
величины r
= п
— k,
то степень многочлена ошибок меньше r.
В этом случае е(Х)
не делится на
(Х)
без остатка и синдром принятого слова
всегда отличен от нулевого, следовательно,
пакет ошибок длины равной или меньшей
r
всегда распознается. Из теоремы 3.6.1
следует, что распознается также любой
циклический сдвиг многочлена В(Х)
степени, меньшей r,
т.е. и «концевой» пакет ошибок длины
меньшей или равной r
(рис. 3.17), всегда распознается.
Теорема 3.7.1.
Циклический (п,
k)-код
способен обнаруживать все пакеты ошибок
(в том числе концевые) длины r
= п
— k
и меньше.
Помимо распознавания
всех пакетов ошибок длины r
и меньше циклические коды обладают
способностью обнаруживать большую
часть пакетов ошибок, длина которых
превосходит r.
Рассмотрим пакет
ошибок длины r
+ 1, начинающийся в j-ой
компоненте. Так как первая и последняя
компоненты пакета ошибок отличны от
нуля, всего имеется 2r-1
возможных конфигураций ошибок.
Необнаружимой является только одна из
них, многочлен которой В(Х)
совпадает с
(Х),
то есть
.
(3.76)
Теорема 3.7.2.
Для циклического (п,
k)-кода
доля необнаружимых пакетов
ошибок длины
l
= r
+ 1 = п
— k+1
равна 2-(r-1).
Рассмотрим пакет
ошибок длины l>r+1=n—k+1
начинающийся в j-ой
компоненте. Если соответствующий
многочлен В(Х)
делится на
(Х)
без остатка, то есть
,
(3.77)
то такой пакет не
может быть обнаружен.
Пусть коэффициенты
многочлена а(Х)
степени l
— r
— 1 имеют вид a0,
a1,
…, al—r1.
Так как пакет ошибок начинается и
заканчивается единицей, a0
= al—r—1.
Следовательно, существует 2l—r—2
наборов коэффициентов а(Х),
приводящих к необнаружимым ошибкам
в (3.77). С другой стороны, существует 2l—2
различных пакетов ошибок длины l
и, таким образом, верно следующее
утверждение.
Теорема 3.7.3.
Для циклического (п,
k)-кода
доля необнаружимых пакетов ошибок длины
l>r
+ l = n
— k
+ 1 равна 2—r.
Пример:
Распознавание ошибок циклическим
(7,4)-кодом Хэмминга.
Рассмотрим
циклический (7,4)-код Хэмминга из предыдущих
примеров с r
= п
— k
= 3. Так как минимальное кодовое расстояние
кода Хэмминга dmin
= 3, он способен обнаруживать все двойные
ошибки или исправлять одиночные.
Рассматриваемый (7,4)-код Хэмминга
является циклическим кодом и он способен
также обнаруживать все пакеты длины r
= 3. В частности, любые три следующие друг
за другом ошибки всегда обнаруживаются.
Доля необнаружимых
ошибок длины r
+ 1 = 4 равна 2-(3-1)
= 1/4. При пакетах ошибок с длиной большей
4, не распознается только 2-3
= 1/8 из них.
Замечание.
На практике,
как правило, используются циклические
коды с довольно большим числом проверочных
разрядов, например, r
= п — k
= 16. Доля необнаружимых пакетов ошибок
такими кодами достаточна мала. Так, при
r
= 16, обнаруживается более чем 99,9969 %
пакетов длины 17 и 99,9984 % пакетов длины
18 и выше.
Соседние файлы в предмете [НЕСОРТИРОВАННОЕ]
- #
- #
- #
- #
- #
- #
- #
- #
- #
- #
- #
Пакет ошибок
Предмет
Информационная безопасность
Разместил
🤓 KerrProsis
👍 Проверено Автор24
комбинация ошибок (как правило, в двоичном сигнале), которая воспринимается как единая ошибка, если ошибочными являются ее определенные элементы («первый» и «последний»), причем промежуточные элементы не обязательно ошибочны. Отсюда следует, что знаки, предшествующие первой ошибке и следующие за последней ошибкой блока, воспринимаются как правильные.
Научные статьи на тему «Пакет ошибок»
LaTeX: возможности расширения и примеры верстки
Для коррекции найденных ошибок необходимо снова вернуться к редактированию исходного TeX -файла, а далее…
снова подвергнуть его компиляции и просмотру на предмет ошибок….
Имя пакета должно быть указано в фигурных скобках….
Пакеты должны состоять из команд TeX, но они не могут генерировать никаких печатных текстов….
Следует отметить, что один и тот же пакет может быть включён в различные тексты.

Статья от экспертов
Точная корректирующая способность кодов Гилберта при исправлении пакетов ошибок
Постановка проблемы: для решения задачи повышения скорости обмена информацией в системах передачи и хранения данных требуется разрабатывать эффективные методы борьбы с помехами, возникающими при передаче, хранении и обработке информации, при использовании как можно меньшей избыточности. Для этого необходимо строить схемы кодирования, ориентированные на ошибки, характерные для конкретного канала связи. Большинство реальных каналов связи являются каналами с группированием ошибок, и типичная помеха в таком канале может описываться как пакет ошибок. Коды Гилберта, обладающие простыми процедурами кодирования и декодирования, хотя и имеют малое минимальное расстояние и неэффективны для исправления независимых ошибок, тем не менее могут быть использованы для исправления пакетов ошибок. Однако корректирующая способность этих кодов при исправлении пакетов ошибок оценивается лишь с помощью не всегда точных границ. Цель: получение точных значений максимальных длин исправляемых пакетов для кодо…
Программные продукты в математическом моделировании
математическое описание модели в программные коды, существует достаточно большая вероятность появления ошибок…
Такие системы иногда именуются просто системами моделирования или пакетами моделирования….
(данный пакет принято называть интерпретирующим)….
Область использования универсальных пакетов значительно более широкая, так как они сориентированы на…
, которые в свою очередь делятся на математические пакеты и пакеты поэлементного моделирования.

Статья от экспертов
Подход к защите информации от пакетов ошибок в средствах радиосвязи декаметрового диапазона
Декаметровая связь, осуществляемая на дальности до сотни километров с помощью мобильных станций небольшой мощности, практически не уступает проводной или радиорелейной. Использование ее актуально не только в мирных, но и в военных целях. Для России связь играет немаловажную роль во многих сферах, а особенно в вопросах развития научного и военного сектора. Ведущие государства стремятся достичь превосходства в околоземном космическом пространстве для развертывания космических группировок способных выполнять широкий спектр задач, но главной из них остается организация связи. В статье проведен анализ особенностей функционирования средств в декаметровом диапазоне, а также рассмотрены способы защиты информации и предложен подход к ее защите от пакетов ошибок.
Повышай знания с онлайн-тренажером от Автор24!
- Напиши термин
- Выбери определение из предложенных или загрузи свое
-
Тренажер от Автор24 поможет тебе выучить термины с помощью удобных и приятных
карточек
пакет ошибок
- пакет ошибок
-
01.02.30 пакет ошибок [ error burst]: Группа битов, в которой два последовательных ошибочных бита всегда разделены правильными битами, число которых менее установленного.
Словарь-справочник терминов нормативно-технической документации.
.
2015.
Смотреть что такое «пакет ошибок» в других словарях:
-
пакет ошибок — — [http://www.iks media.ru/glossary/index.html?glossid=2400324] Тематики электросвязь, основные понятия EN burst error … Справочник технического переводчика
-
пакет ошибок — klaidų paketas statusas T sritis automatika atitikmenys: angl. burst error; cluster of errors vok. Fehlerhäufung, f rus. пакет ошибок, m; пачка ошибок, f pranc. groupe d erreurs, m … Automatikos terminų žodynas
-
одиночный пакет ошибок — — [Л.Г.Суменко. Англо русский словарь по информационным технологиям. М.: ГП ЦНИИС, 2003.] Тематики информационные технологии в целом EN single burst of errors … Справочник технического переводчика
-
плотный пакет ошибок — — [Л.Г.Суменко. Англо русский словарь по информационным технологиям. М.: ГП ЦНИИС, 2003.] Тематики информационные технологии в целом EN solid burst error … Справочник технического переводчика
-
пакет ошибочных единичных элементов цифрового сигнала данных — пакет ошибок Группа ошибочных единичных элементов цифрового сигнала данных, начинающаяся и кончающаяся ошибочными единичными элементами этого сигнала, в которой число правильных единичных элементов, разделяющих два соседних ошибочных единичных… … Справочник технического переводчика
-
пакет — 01.02.04 пакет [ packet] (1): Блок данных, посланный по каналу связи. Примечание Каждый пакет может содержать в дополнение к фактическому сообщению информацию об отправителе, получателе, а также для контроля ошибок. Пакеты могут иметь… … Словарь-справочник терминов нормативно-технической документации
-
Пакет ошибочных единичных элементов цифрового сигнала данных — 80. Пакет ошибочных единичных элементов цифрового сигнала данных Пакет ошибок Е. Erroneous element burst Группа ошибочных единичных элементов цифрового сигнала данных, начинающаяся и кончающаяся ошибочными единичными элементами этого сигнала, в… … Словарь-справочник терминов нормативно-технической документации
-
Пакет обновления — (от английского service pack, сокращенно SP) набор обновлений, исправлений и/или улучшений компьютерной программы, поставляемый в виде единого установочного пакета. Многие компании, как например, Microsoft или Autodesk, обычно выпускают пакет… … Википедия
-
пакет обновления ПО — Комплект исправленных ошибок и дополнений для программного продукта, оформленный в виде установочного пакета. Microsoft регулярно выпускает пакеты обновлений для Windows и других приложений. [http://www.morepc.ru/dict/] Тематики информационные… … Справочник технического переводчика
-
Пакет (сетевые технологии) — В компьютерных сетях пакет это определённым образом оформленный блок данных, передаваемый по сети в пакетном режиме. Компьютерные линии связи, которые не поддерживают пакетный режим, как, например, традиционная телекоммуникационная связь… … Википедия
In coding theory, burst error-correcting codes employ methods of correcting burst errors, which are errors that occur in many consecutive bits rather than occurring in bits independently of each other.
Many codes have been designed to correct random errors. Sometimes, however, channels may introduce errors which are localized in a short interval. Such errors occur in a burst (called burst errors) because they occur in many consecutive bits. Examples of burst errors can be found extensively in storage mediums. These errors may be due to physical damage such as scratch on a disc or a stroke of lightning in case of wireless channels. They are not independent; they tend to be spatially concentrated. If one bit has an error, it is likely that the adjacent bits could also be corrupted. The methods used to correct random errors are inefficient to correct burst errors.
Definitions[edit]
A burst of length ℓ[1]
Say a codeword 







Although this definition is sufficient to describe what a burst error is, the majority of the tools developed for burst error correction rely on cyclic codes. This motivates our next definition.
A cyclic burst of length ℓ[1]
An error vector 








For the remainder of this article, we will use the term burst to refer to a cyclic burst, unless noted otherwise.
Burst description[edit]
It is often useful to have a compact definition of a burst error, that encompasses not only its length, but also the pattern, and location of such error. We define a burst description to be a tuple 


For example, the burst description of the error pattern 







Definition. The number of symbols in a given error pattern 

A corollary of the above theorem is that we cannot have two distinct burst descriptions for bursts of length 
Cyclic codes for burst error correction[edit]
Cyclic codes are defined as follows: think of the 


Codewords are polynomials of degree 








Cyclic codes can detect all bursts of length up to 


Theorem (Cyclic burst correction capability) — Every cyclic code with generator polynomial of degree 

The above proof suggests a simple algorithm for burst error detection/correction in cyclic codes: given a transmitted word (i.e. a polynomial of degree 



By the upper bound on burst error detection (









We now consider a fundamental theorem about cyclic codes that will aid in designing efficient burst-error correcting codes, by categorizing bursts into different cosets.
Burst error correction bounds[edit]
Upper bounds on burst error detection and correction[edit]
By upper bound, we mean a limit on our error detection ability that we can never go beyond. Suppose that we want to design an 






Theorem (Burst error detection ability) — The burst error detection ability of any 

Now, we repeat the same question but for error correction: given 



Theorem (Burst error correction ability) — The burst error correction ability of any 

A stronger result is given by the Rieger bound:
Definition. A linear burst-error-correcting code achieving the above Rieger bound is called an optimal burst-error-correcting code.
Further bounds on burst error correction[edit]
There is more than one upper bound on the achievable code rate of linear block codes for multiple phased-burst correction (MPBC). One such bound is constrained to a maximum correctable cyclic burst length within every subblock, or equivalently a constraint on the minimum error free length or gap within every phased-burst. This bound, when reduced to the special case of a bound for single burst correction, is the Abramson bound (a corollary of the Hamming bound for burst-error correction) when the cyclic burst length is less than half the block length.[3]
Theorem (Abramson’s bounds) — If 

Proof
For a linear 

Isolating 




Remark. 

Fire codes[3][4][5][edit]
While cyclic codes in general are powerful tools for detecting burst errors, we now consider a family of binary cyclic codes named Fire Codes, which possess good single burst error correction capabilities. By single burst, say of length 

Let 














We will show that 

Lemma 1 — 
Lemma 2 — If 



Proof
If 


Now suppose 








But 








A corollary to Lemma 2 is that since 




Theorem — The Fire Code is 
If we can show that all bursts of length 
Proof of Theorem[edit]
Let 















Notice that at the second manipulation, we introduced the term 














Then we may write:
Equating the degree of both sides, gives us 




The term 












Since 


















Example: 5-burst error correcting fire code[edit]
With the theory presented in the above section, consider the construction of a 










is a Fire Code generator. We can calculate the block-length of the code by evaluating the least common multiple of 



Binary Reed–Solomon codes[edit]
Certain families of codes, such as Reed–Solomon, operate on alphabet sizes larger than binary. This property awards such codes powerful burst error correction capabilities. Consider a code operating on 





![{displaystyle [mn,mk]_{2}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/479cc66e2a601a8f28c80c0d3136e9d45f84728d)

The reason such codes are powerful for burst error correction is that each symbol is represented by 


Notice that a burst of 







In general, a 

or fewer bursts of length 

An example of a binary RS code[edit]
Let 
![{displaystyle [255,223,33]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bc05fee57b496252bf9064f1e35606fae8d805dc)





![{displaystyle [2040,1784,33]_{2}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e0de5be730230c649eac90e01bb084dbcd81319a)

Interleaved codes[edit]
Interleaving is used to convert convolutional codes from random error correctors to burst error correctors. The basic idea behind the use of interleaved codes is to jumble symbols at the transmitter. This leads to randomization of bursts of received errors which are closely located and we can then apply the analysis for random channel. Thus, the main function performed by the interleaver at transmitter is to alter the input symbol sequence. At the receiver, the deinterleaver will alter the received sequence to get back the original unaltered sequence at the transmitter.
Burst error correcting capacity of interleaver[edit]
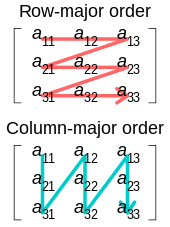
Illustration of row- and column-major order
Block interleaver[edit]
The figure below shows a 4 by 3 interleaver.
An example of a block interleaver
The above interleaver is called as a block interleaver. Here, the input symbols are written sequentially in the rows and the output symbols are obtained by reading the columns sequentially. Thus, this is in the form of 

Capacity of block interleaver: For an 






Efficiency of block interleaver (

Drawbacks of block interleaver : As it is clear from the figure, the columns are read sequentially, the receiver can interpret single row only after it receives complete message and not before that. Also, the receiver requires a considerable amount of memory in order to store the received symbols and has to store the complete message. Thus, these factors give rise to two drawbacks, one is the latency and other is the storage (fairly large amount of memory). These drawbacks can be avoided by using the convolutional interleaver described below.
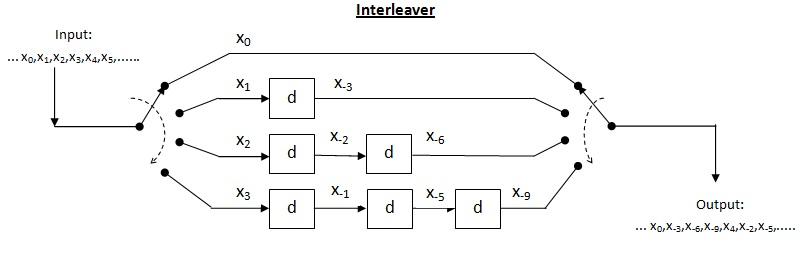
Convolutional interleaver[edit]
Cross interleaver is a kind of multiplexer-demultiplexer system. In this system, delay lines are used to progressively increase length. Delay line is basically an electronic circuit used to delay the signal by certain time duration. Let 









An example of a convolutional interleaver
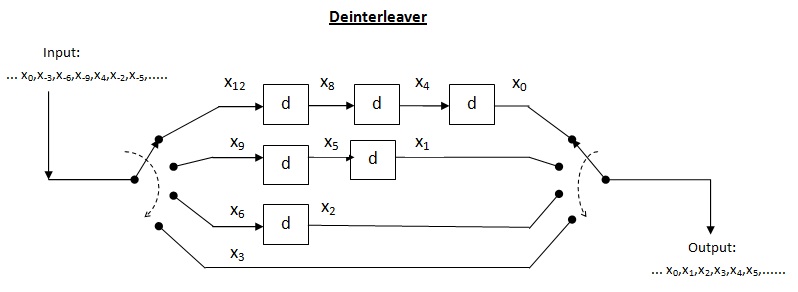
An example of a deinterleaver
Efficiency of cross interleaver (
Thus, we can formulate 
Performance of cross interleaver : As shown in the above interleaver figure, the output is nothing but the diagonal symbols generated at the end of each delay line. In this case, when the input multiplexer switch completes around half switching, we can read first row at the receiver. Thus, we need to store maximum of around half message at receiver in order to read first row. This drastically brings down the storage requirement by half. Since just half message is now required to read first row, the latency is also reduced by half which is good improvement over the block interleaver. Thus, the total interleaver memory is split between transmitter and receiver.
Applications[edit]
Compact disc[edit]
Without error correcting codes, digital audio would not be technically feasible.[7] The Reed–Solomon codes can correct a corrupted symbol with a single bit error just as easily as it can correct a symbol with all bits wrong. This makes the RS codes particularly suitable for correcting burst errors.[5] By far, the most common application of RS codes is in compact discs. In addition to basic error correction provided by RS codes, protection against burst errors due to scratches on the disc is provided by a cross interleaver.[3]
Current compact disc digital audio system was developed by N. V. Philips of The Netherlands and Sony Corporation of Japan (agreement signed in 1979).
A compact disc comprises a 120 mm aluminized disc coated with a clear plastic coating, with spiral track, approximately 5 km in length, which is optically scanned by a laser of wavelength ~0.8 μm, at a constant speed of ~1.25 m/s. For achieving this constant speed, rotation of the disc is varied from ~8 rev/s while scanning at the inner portion of the track to ~3.5 rev/s at the outer portion. Pits and lands are the depressions (0.12 μm deep) and flat segments constituting the binary data along the track (0.6 μm width).[8]
The CD process can be abstracted as a sequence of the following sub-processes:
- Channel encoding of source of signals
- Mechanical sub-processes of preparing a master disc, producing user discs and sensing the signals embedded on user discs while playing – the channel
- Decoding the signals sensed from user discs
The process is subject to both burst errors and random errors.[7] Burst errors include those due to disc material (defects of aluminum reflecting film, poor reflective index of transparent disc material), disc production (faults during disc forming and disc cutting etc.), disc handling (scratches – generally thin, radial and orthogonal to direction of recording) and variations in play-back mechanism. Random errors include those due to jitter of reconstructed signal wave and interference in signal. CIRC (Cross-Interleaved Reed–Solomon code) is the basis for error detection and correction in the CD process. It corrects error bursts up to 3,500 bits in sequence (2.4 mm in length as seen on CD surface) and compensates for error bursts up to 12,000 bits (8.5 mm) that may be caused by minor scratches.
Encoding: Sound-waves are sampled and converted to digital form by an A/D converter. The sound wave is sampled for amplitude (at 44.1 kHz or 44,100 pairs, one each for the left and right channels of the stereo sound). The amplitude at an instance is assigned a binary string of length 16. Thus, each sample produces two binary vectors from 

Input for the encoder consists of input frames each of 24 8-bit symbols (12 16-bit samples from the A/D converter, 6 each from left and right data (sound) sources). A frame can be represented by 



Initially, the bytes are permuted to form new frames represented by 


Next, these 24 message symbols are encoded using C2 (28,24,5) Reed–Solomon code which is a shortened RS code over 



Decoding: The CD player (CIRC decoder) receives the 32 output symbol data stream. This stream passes through the decoder D1 first. It is up to individual designers of CD systems to decide on decoding methods and optimize their product performance. Being of minimum distance 5 The D1, D2 decoders can each correct a combination of 


Performance of CIRC:[7] CIRC conceals long bust errors by simple linear interpolation. 2.5 mm of track length (4000 bits) is the maximum completely correctable burst length. 7.7 mm track length (12,300 bits) is the maximum burst length that can be interpolated. Sample interpolation rate is one every 10 hours at Bit Error Rate (BER) 



See also[edit]
- Error detection and correction
- Error-correcting codes with feedback
- Code rate
- Reed–Solomon error correction
References[edit]
- ^ a b c d Coding Bounds for Multiple Phased-Burst Correction and Single Burst Correction Codes
- ^ The Theory of Information and Coding: Student Edition, by R. J. McEliece
- ^ a b c Ling, San, and Chaoping Xing. Coding Theory: A First Course. Cambridge, UK: Cambridge UP, 2004. Print
- ^ a b Moon, Todd K. Error Correction Coding: Mathematical Methods and Algorithms. Hoboken, NJ: Wiley-Interscience, 2005. Print
- ^ a b c d e f Lin, Shu, and Daniel J. Costello. Error Control Coding: Fundamentals and Applications. Upper Saddle River, NJ: Pearson-Prentice Hall, 2004. Print
- ^ quest.arc.nasa.gov https://web.archive.org/web/20120627022807/http://quest.arc.nasa.gov/saturn/qa/cassini/Error_correction.txt. Archived from the original on 2012-06-27.
- ^ a b c Algebraic Error Control Codes (Autumn 2012) – Handouts from Stanford University
- ^ McEliece, Robert J. The Theory of Information and Coding: A Mathematical Framework for Communication. Reading, MA: Addison-Wesley Pub., Advanced Book Program, 1977. Print
Сразу хочу сказать, что здесь никакой воды про циклические коды исправляющие пакеты ошибок , и только нужная информация. Для того чтобы лучше понимать что такое
циклические коды исправляющие пакеты ошибок , настоятельно рекомендую прочитать все из категории Теория информации и кодирования.
Циклические коды, исправляющие пакеты ошибок.
В общем случае любой корректирующий код исправляющий t ошибок исправляет любую конфигурацию из t ошибок. Вместе с тем, если заранее известно, что ошибки расположены пакетом, то можно сконструировать коды более эффективно. Пакет ошибок описывается в виде e(x)=xi*b(x)(mod xn-1), где b(x) — многочлен, степень которого не выше чем t-1, xi — локатор пакета, i — номер разряда.
Синдромные многочлены S(x) для исправляющего пакеты ошибок ЦК должны быть различны для любого пакета длины не более t.
Пример: g(x)=x6+ x3+ x2+ x+1, n=15 и корректирует пакеты из трех и менее ошибок.
e(x)=xi,i=0,…,14
e(x)=xi(1+x)(mod x15-1)
e(x)=xi(1+x2)(mod x15-1)
e(x)=xi(1+x+x2)(mod x15-1)
Непосредственным вычислением проявляется, что синдромы для всех 56 возможных пакетов различны . Об этом говорит сайт https://intellect.icu . Следовательно, g(x)=x6+ x3+ x2+ x+1 порождает код, исправляющий все пакеты длины 3.
Как правило, ЦК, исправляющие пакеты ошибок синтезируются с помощью ЭВМ.
Статью про циклические коды исправляющие пакеты ошибок я написал специально для тебя. Если ты хотел бы внести свой вклад в развии теории и практики,
ты можешь написать коммент или статью отправив на мою почту в разделе контакты.
Этим ты поможешь другим читателям, ведь ты хочешь это сделать? Надеюсь, что теперь ты понял что такое циклические коды исправляющие пакеты ошибок
и для чего все это нужно, а если не понял, или есть замечания,
то нестесняся пиши или спрашивай в комментариях, с удовольствием отвечу. Для того чтобы глубже понять настоятельно рекомендую изучить всю информацию из категории
Теория информации и кодирования
Из статьи мы узнали кратко, но емко про циклические коды исправляющие пакеты ошибок
В последнее десятилетие мы успешно пользовались тем, что Go обрабатывает ошибки как значения. Хотя в стандартной библиотеке была минимальная поддержка ошибок: лишь функции errors.New и fmt.Errorf, которые генерируют ошибку, содержащую только сообщение — встроенный интерфейс позволяет Go-программистам добавлять любую информацию. Нужен лишь тип, реализующий метод Error:
type QueryError struct {
Query string
Err error
}
func (e *QueryError) Error() string { return e.Query + ": " + e.Err.Error() }Такие типы ошибок встречаются во всех языках и хранят самую разную информацию, от временных меток до названий файлов и адресов серверов. Часто упоминаются и низкоуровневые ошибки, предоставляющие дополнительный контекст.
Паттерн, когда одна ошибка содержит другую, встречается в Go столь часто, что после жаркой дискуссии в Go 1.13 была добавлена его явная поддержка. В этой статье мы рассмотрим дополнения к стандартной библиотеке, обеспечивающие упомянутую поддержку: три новые функции в пакете errors и новая форматирующая команда для fmt.Errorf.
Прежде чем подробно рассматривать изменения, давайте поговорим о том, как ошибки исследовались и конструировались в предыдущих версиях языка.
Ошибки до Go 1.13
Исследование ошибок
Ошибки в Go являются значениями. Программы принимают решения на основе этих значений разными способами. Чаще всего ошибка сравнивается с nil, чтобы понять, не было ли сбоя операции.
if err != nil {
// something went wrong
}
Иногда мы сравниваем ошибку, чтобы узнать контрольное значение и понять, не возникла ли конкретная ошибка.
var ErrNotFound = errors.New("not found")
if err == ErrNotFound {
// something wasn't found
}Значение-ошибка может быть любого типа, который удовлетворяет определённому в языке интерфейсу ошибок. Программа может использовать утверждение типа или переключатель типа для просмотра значения-ошибки более специфического типа.
type NotFoundError struct {
Name string
}
func (e *NotFoundError) Error() string { return e.Name + ": not found" }
if e, ok := err.(*NotFoundError); ok {
// e.Name wasn't found
}
Добавление информации
Зачастую функция передаёт ошибку вверх по стеку вызовов, добавляя к ней информацию, например, короткое описание того, что происходило в момент возникновения ошибки. Это сделать просто, достаточно сконструировать новую ошибку, включающую в себя текст из предыдущей ошибки:
if err != nil {
return fmt.Errorf("decompress %v: %v", name, err)
}
При создании новой ошибки с помощью fmt.Errorf мы выбрасываем из исходной ошибки всё, за исключением текста. Как мы видели в примере с QueryError, иногда нужно определять новый тип ошибки, который содержит исходную ошибку, чтобы сохранить её для анализа с помощью кода:
type QueryError struct {
Query string
Err error
}
Программы могут заглянуть внутрь значения *QueryError и принять решение на основе исходной ошибки. Иногда это называется «распаковкой» (unwrapping) ошибки.
if e, ok := err.(*QueryError); ok && e.Err == ErrPermission {
// query failed because of a permission problem
}
Тип os.PathError из стандартной библиотеки — ещё пример того, как одна ошибка содержит другую.
Ошибки в Go 1.13
Метод Unwrap
В Go 1.13 в пакетах стандартной библиотеки errors и fmt упрощена работа с ошибками, которые содержат другие ошибки. Самым важным является соглашение, а не изменение: ошибка, содержащая другую ошибку, может реализовать метод Unwrap, который возвращает исходную ошибку. Если e1.Unwrap() возвращает e2, то мы говорим, что e1 упаковывает e2 и можно распаковать e1 для получения e2.
Согласно этому соглашению, можно дать описанный выше тип QueryError методу Unwrap, который возвращает содержащуюся в нём ошибку:
func (e *QueryError) Unwrap() error { return e.Err }
Результат распаковки ошибки тоже может содержать метод Unwrap. Последовательность ошибок, полученных с помощью повторяющихся распаковок, мы называем цепочкой ошибок.
Исследование ошибок с помощью Is и As
В Go 1.13 пакет errors содержит две новые функции для исследования ошибок: Is и As.
Функция errors.Is сравнивает ошибку со значением.
// Similar to:
// if err == ErrNotFound { … }
if errors.Is(err, ErrNotFound) {
// something wasn't found
}
Функция As проверяет, относится ли ошибка к конкретному типу.
// Similar to:
// if e, ok := err.(*QueryError); ok { … }
var e *QueryError
if errors.As(err, &e) {
// err is a *QueryError, and e is set to the error's value
}
В простейшем случае функция errors.Is ведёт себя как сравнение с контрольной ошибкой, а функция errors.As ведёт себя как утверждение типа. Однако работая с упакованными ошибками, эти функции оценивают все ошибки в цепочке. Давайте посмотрим на вышеприведённый пример распаковки QueryError для исследования исходной ошибки:
if e, ok := err.(*QueryError); ok && e.Err == ErrPermission {
// query failed because of a permission problem
}
С помощью функции errors.Is можно записать так:
if errors.Is(err, ErrPermission) {
// err, or some error that it wraps, is a permission problem
}
Пакет errors также содержит новую функцию Unwrap, которая возвращает результат вызова метода Unwrap ошибки, или возвращает nil, если у ошибки нет метода Unwrap. Обычно лучше использовать errors.Is или errors.As, поскольку они позволяют исследовать всю цепочку одним вызовом.
Упаковка ошибок с помощью %w
Как я упоминал, нормальной практикой является использование функции fmt.Errorf для добавления к ошибке дополнительной информации.
if err != nil {
return fmt.Errorf("decompress %v: %v", name, err)
}
В Go 1.13 функция fmt.Errorf поддерживает новая команда %w. Если она есть, то ошибка, возвращаемая fmt.Errorf, будет содержать метод Unwrap, возвращающий аргумент %w, который должен быть ошибкой. Во всех остальных случаях %w идентична %v.
if err != nil {
// Return an error which unwraps to err.
return fmt.Errorf("decompress %v: %w", name, err)
}
Упаковка ошибки с помощью %w делает её доступной для errors.Is и errors.As:
err := fmt.Errorf("access denied: %w", ErrPermission)
...
if errors.Is(err, ErrPermission) ...
Когда стоит упаковывать?
Когда вы добавляете к ошибке дополнительный контекст с помощью fmt.Errorf или реализации пользовательского типа вам нужно решить, будет ли новая ошибка содержать в себе исходную. На это нет однозначного ответа, всё зависит от контекста, в котором создана новая ошибка. Упакуйте, чтобы показать её вызывающим. Не упаковывайте ошибку, если это приведёт к раскрытию подробностей реализации.
Например, представьте функцию Parse, которая считывает из io.Reader сложную структуру данных. Если возникает ошибка, нам захочется узнать номер строки и столбца, где она произошла. Если ошибка возникла при чтении из io.Reader, нам нужно будет упаковать её, чтобы выяснить причину. Поскольку вызывающий был предоставлен функции io.Reader, имеет смысл показать сгенерированную им ошибку.
Другой случай: функция, которая делает несколько вызовов базы данных, вероятно, не должна возвращать ошибку, в которой упакован результат одного из этих вызовов. Если БД, которая использовалась этой функцией, является частью реализации, то раскрытие этих ошибок нарушит абстракцию. К примеру, если функция LookupUser из пакета pkg использует пакет Go database/sql, то она может столкнуться с ошибкой sql.ErrNoRows. Если вернуть ошибку с помощью fmt.Errorf("accessing DB: %v", err), тогда вызывающий не может заглянуть внутрь и найти sql.ErrNoRows. Но если функция вернёт fmt.Errorf("accessing DB: %w", err), тогда вызывающий мог бы написать:
err := pkg.LookupUser(...)
if errors.Is(err, sql.ErrNoRows) …
В таком случае функция должна всегда возвращать sql.ErrNoRows, если вы не хотите сломать клиенты, даже при переключении на пакет с другой базой данных. Иными словами, упаковка делает ошибку частью вашего API. Если не хотите в будущем коммитить поддержку этой ошибки как часть API, не упаковывайте её.
Важно помнить, что вне зависимости от того, упаковываете вы её или нет, ошибка останется неизменной. Человек, который будет в ней разбираться, будет иметь одну и ту же информацию. Принятие решения об упаковке зависит от того, нужно ли дать дополнительную информацию программам, чтобы они могли принимать более информированные решения; или если нужно скрыть эту информацию ради сохранения уровня абстракции.
Настройка тестирования ошибок с помощью методов Is и As
Функция errors.Is проверяет каждую ошибку в цепочке на соответствие целевому значению. По умолчанию ошибка соответствует этому значению, если они эквивалентны. Кроме того, ошибка в цепочке может объявлять о своём соответствии целевому значению с помощью реализации метода Is.
Рассмотрим ошибку, вызванную пакетом Upspin, которая сравнивает ошибку с шаблоном и оценивает только ненулевые поля:
type Error struct {
Path string
User string
}
func (e *Error) Is(target error) bool {
t, ok := target.(*Error)
if !ok {
return false
}
return (e.Path == t.Path || t.Path == "") &&
(e.User == t.User || t.User == "")
}
if errors.Is(err, &Error{User: "someuser"}) {
// err's User field is "someuser".
}
Функция errors.As также консультирует метод As при его наличии.
Ошибки и API пакетов
Пакет, который возвращает ошибки (а это делают большинство пакетов), должен описать свойства этих ошибок, на которые может опираться программист. Хорошо спроектированный пакет также будет избегать возвращения ошибок со свойствами, на которые нельзя опираться.
Самое простое: говорить, была ли операция успешной, возвращая, соответственно, значение nil или не-nil. Во многих случаях другой информации не требуется.
Если вам нужно, чтобы функция возвращала индентифицируемое состояние ошибки, например, «элемент не найден», то можно возвращать ошибку, в которую упаковано сигнальное значение.
var ErrNotFound = errors.New("not found")
// FetchItem returns the named item.
//
// If no item with the name exists, FetchItem returns an error
// wrapping ErrNotFound.
func FetchItem(name string) (*Item, error) {
if itemNotFound(name) {
return nil, fmt.Errorf("%q: %w", name, ErrNotFound)
}
// ...
}Есть и другие паттерны предоставления ошибок, которые вызывающий может семантически изучить. Например, напрямую возвращать контрольное значение, конкретный тип, или значение, которое можно проанализировать с помощью предикативной функции.
В любом случае, не раскрывайте пользователю внутренние подробности. Как упоминалось в главе «Когда стоит упаковывать?», если возвращаете ошибку из другого пакета, то преобразуйте её, чтобы не раскрывать исходную ошибку, если только не собираетесь брать на себя обязательство в будущем вернуть эту конкретную ошибку.
f, err := os.Open(filename)
if err != nil {
// The *os.PathError returned by os.Open is an internal detail.
// To avoid exposing it to the caller, repackage it as a new
// error with the same text. We use the %v formatting verb, since
// %w would permit the caller to unwrap the original *os.PathError.
return fmt.Errorf("%v", err)
}Если функция возвращает ошибку с упакованным сигнальным значением или типом, то не возвращайте напрямую исходную ошибку.
var ErrPermission = errors.New("permission denied")
// DoSomething returns an error wrapping ErrPermission if the user
// does not have permission to do something.
func DoSomething() {
if !userHasPermission() {
// If we return ErrPermission directly, callers might come
// to depend on the exact error value, writing code like this:
//
// if err := pkg.DoSomething(); err == pkg.ErrPermission { … }
//
// This will cause problems if we want to add additional
// context to the error in the future. To avoid this, we
// return an error wrapping the sentinel so that users must
// always unwrap it:
//
// if err := pkg.DoSomething(); errors.Is(err, pkg.ErrPermission) { ... }
return fmt.Errorf("%w", ErrPermission)
}
// ...
}
Заключение
Хотя мы обсудили всего лишь три функции и форматирующую команду, надеемся, что они помогут сильно улучшить обработку ошибок в программах на Go. Мы надеемся, что упаковка ради предоставления дополнительного контекста станет нормальной практикой, помогающей программистам принимать более взвешенные решения и быстрее находить баги.
Как сказал Расс Кокс (Russ Cox) в своём выступлении на GopherCon 2019, на пути к Go 2 мы экспериментируем, упрощаем и отгружаем. И теперь, отгрузив эти изменения, мы принимаемся за новые эксперименты.