Ошибка игрока ложный вывод монте карло

The gambler’s fallacy, also known as the Monte Carlo fallacy or the fallacy of the maturity of chances, is the incorrect belief that, if a particular event occurs more frequently than normal during the past, it is less likely to happen in the future (or vice versa), when it has otherwise been established that the probability of such events does not depend on what has happened in the past. Such events, having the quality of historical independence, are referred to as statistically independent. The fallacy is commonly associated with gambling, where it may be believed, for example, that the next dice roll is more than usually likely to be six because there have recently been fewer than the expected number of sixes.

The term «Monte Carlo fallacy» originates from the best known example of the phenomenon, which occurred in the Monte Carlo Casino in 1913.^[1]

Examples[edit]

Coin toss[edit]

The gambler’s fallacy can be illustrated by considering the repeated toss of a fair coin. The outcomes in different tosses are statistically independent and the probability of getting heads on a single toss is 1/2 (one in two). The probability of getting two heads in two tosses is 1/4 (one in four) and the probability of getting three heads in three tosses is 1/8 (one in eight). In general, if A_i is the event where toss i of a fair coin comes up heads, then:

.

If after tossing four heads in a row, the next coin toss also came up heads, it would complete a run of five successive heads. Since the probability of a run of five successive heads is 1/32 (one in thirty-two), a person might believe that the next flip would be more likely to come up tails rather than heads again. This is incorrect and is an example of the gambler’s fallacy. The event «5 heads in a row» and the event «first 4 heads, then a tails» are equally likely, each having probability 1/32. Since the first four tosses turn up heads, the probability that the next toss is a head is:

.

While a run of five heads has a probability of 1/32 = 0.03125 (a little over 3%), the misunderstanding lies in not realizing that this is the case only before the first coin is tossed. After the first four tosses in this example, the results are no longer unknown, so their probabilities are at that point equal to 1 (100%). The probability of a run of coin tosses of any length continuing for one more toss is always 0.5. The reasoning that a fifth toss is more likely to be tails because the previous four tosses were heads, with a run of luck in the past influencing the odds in the future, forms the basis of the fallacy.

Why the probability is 1/2 for a fair coin[edit]

If a fair coin is flipped 21 times, the probability of 21 heads is 1 in 2,097,152. The probability of flipping a head after having already flipped 20 heads in a row is 1/2. Assuming a fair coin:

• The probability of 20 heads, then 1 tail is 0.5²⁰ × 0.5 = 0.5²¹
• The probability of 20 heads, then 1 head is 0.5²⁰ × 0.5 = 0.5²¹

The probability of getting 20 heads then 1 tail, and the probability of getting 20 heads then another head are both 1 in 2,097,152. When flipping a fair coin 21 times, the outcome is equally likely to be 21 heads as 20 heads and then 1 tail. These two outcomes are equally as likely as any of the other combinations that can be obtained from 21 flips of a coin. All of the 21-flip combinations will have probabilities equal to 0.5²¹, or 1 in 2,097,152. Assuming that a change in the probability will occur as a result of the outcome of prior flips is incorrect because every outcome of a 21-flip sequence is as likely as the other outcomes. In accordance with Bayes’ theorem, the likely outcome of each flip is the probability of the fair coin, which is 1/2.

Other examples[edit]

The fallacy leads to the incorrect notion that previous failures will create an increased probability of success on subsequent attempts. For a fair 16-sided die, the probability of each outcome occurring is 1/16 (6.25%). If a win is defined as rolling a 1, the probability of a 1 occurring at least once in 16 rolls is:

The probability of a loss on the first roll is 15/16 (93.75%). According to the fallacy, the player should have a higher chance of winning after one loss has occurred. The probability of at least one win is now:

By losing one toss, the player’s probability of winning drops by two percentage points. With 5 losses and 11 rolls remaining, the probability of winning drops to around 0.5 (50%). The probability of at least one win does not increase after a series of losses; indeed, the probability of success actually decreases, because there are fewer trials left in which to win. The probability of winning will eventually be equal to the probability of winning a single toss, which is 1/16 (6.25%) and occurs when only one toss is left.

Reverse position[edit]

After a consistent tendency towards tails, a gambler may also decide that tails has become a more likely outcome. This is a rational and Bayesian conclusion, bearing in mind the possibility that the coin may not be fair; it is not a fallacy. Believing the odds to favor tails, the gambler sees no reason to change to heads. However it is a fallacy that a sequence of trials carries a memory of past results which tend to favor or disfavor future outcomes.

The inverse gambler’s fallacy described by Ian Hacking is a situation where a gambler entering a room and seeing a person rolling a double six on a pair of dice may erroneously conclude that the person must have been rolling the dice for quite a while, as they would be unlikely to get a double six on their first attempt.

Retrospective gambler’s fallacy[edit]

Researchers have examined whether a similar bias exists for inferences about unknown past events based upon known subsequent events, calling this the «retrospective gambler’s fallacy».^[2]

An example of a retrospective gambler’s fallacy would be to observe multiple successive «heads» on a coin toss and conclude from this that the previously unknown flip was «tails».^[2] Real world examples of retrospective gambler’s fallacy have been argued to exist in events such as the origin of the Universe. In his book Universes, John Leslie argues that «the presence of vastly many universes very different in their characters might be our best explanation for why at least one universe has a life-permitting character».^[3] Daniel M. Oppenheimer and Benoît Monin argue that «In other words, the ‘best explanation’ for a low-probability event is that it is only one in a multiple of trials, which is the core intuition of the reverse gambler’s fallacy.»^[2] Philosophical arguments are ongoing about whether such arguments are or are not a fallacy, arguing that the occurrence of our universe says nothing about the existence of other universes or trials of universes.^[4][5] Three studies involving Stanford University students tested the existence of a retrospective gamblers’ fallacy. All three studies concluded that people have a gamblers’ fallacy retrospectively as well as to future events.^[2] The authors of all three studies concluded their findings have significant «methodological implications» but may also have «important theoretical implications» that need investigation and research, saying «[a] thorough understanding of such reasoning processes requires that we not only examine how they influence our predictions of the future, but also our perceptions of the past.»^[2]

Childbirth[edit]

In 1796, Pierre-Simon Laplace described in A Philosophical Essay on Probabilities the ways in which men calculated their probability of having sons: «I have seen men, ardently desirous of having a son, who could learn only with anxiety of the births of boys in the month when they expected to become fathers. Imagining that the ratio of these births to those of girls ought to be the same at the end of each month, they judged that the boys already born would render more probable the births next of girls.» The expectant fathers feared that if more sons were born in the surrounding community, then they themselves would be more likely to have a daughter. This essay by Laplace is regarded as one of the earliest descriptions of the fallacy.^[6] Likewise, after having multiple children of the same sex, some parents may erroneously believe that they are due to have a child of the opposite sex.

Monte Carlo Casino[edit]

Perhaps the most famous example of the gambler’s fallacy occurred in a game of roulette at the Monte Carlo Casino on August 18, 1913, when the ball fell in black 26 times in a row. This was an extremely uncommon occurrence: the probability of a sequence of either red or black occurring 26 times in a row is (18/37)^26-1 or around 1 in 66.6 million, assuming the mechanism is unbiased. Gamblers lost millions of francs betting against black, reasoning incorrectly that the streak was causing an imbalance in the randomness of the wheel, and that it had to be followed by a long streak of red.^[1]

Non-examples[edit]

Non-independent events[edit]

The gambler’s fallacy does not apply when the probability of different events is not independent. In such cases, the probability of future events can change based on the outcome of past events, such as the statistical permutation of events. An example is when cards are drawn from a deck without replacement. If an ace is drawn from a deck and not reinserted, the next card drawn is less likely to be an ace and more likely to be of another rank. The probability of drawing another ace, assuming that it was the first card drawn and that there are no jokers, has decreased from 4/52 (7.69%) to 3/51 (5.88%), while the probability for each other rank has increased from 4/52 (7.69%) to 4/51 (7.84%). This effect allows card counting systems to work in games such as blackjack.

Bias[edit]

In most illustrations of the gambler’s fallacy and the reverse gambler’s fallacy, the trial (e.g. flipping a coin) is assumed to be fair. In practice, this assumption may not hold. For example, if a coin is flipped 21 times, the probability of 21 heads with a fair coin is 1 in 2,097,152. Since this probability is so small, if it happens, it may well be that the coin is somehow biased towards landing on heads, or that it is being controlled by hidden magnets, or similar.^[7] In this case, the smart bet is «heads» because Bayesian inference from the empirical evidence — 21 heads in a row — suggests that the coin is likely to be biased toward heads. Bayesian inference can be used to show that when the long-run proportion of different outcomes is unknown but exchangeable (meaning that the random process from which the outcomes are generated may be biased but is equally likely to be biased in any direction) and that previous observations demonstrate the likely direction of the bias, the outcome which has occurred the most in the observed data is the most likely to occur again.^[8]

For example, if the a priori probability of a biased coin is say 1%, and assuming that such a biased coin would come down heads say 60% of the time, then after 21 heads the probability of a biased coin has increased to about 32%.

The opening scene of the play Rosencrantz and Guildenstern Are Dead by Tom Stoppard discusses these issues as one man continually flips heads and the other considers various possible explanations.

Changing probabilities[edit]

If external factors are allowed to change the probability of the events, the gambler’s fallacy may not hold. For example, a change in the game rules might favour one player over the other, improving his or her win percentage. Similarly, an inexperienced player’s success may decrease after opposing teams learn about and play against their weaknesses. This is another example of bias.

Psychology[edit]

Origins[edit]

The gambler’s fallacy arises out of a belief in a law of small numbers, leading to the erroneous belief that small samples must be representative of the larger population. According to the fallacy, streaks must eventually even out in order to be representative.^[9] Amos Tversky and Daniel Kahneman first proposed that the gambler’s fallacy is a cognitive bias produced by a psychological heuristic called the representativeness heuristic, which states that people evaluate the probability of a certain event by assessing how similar it is to events they have experienced before, and how similar the events surrounding those two processes are.^[10][9] According to this view, «after observing a long run of red on the roulette wheel, for example, most people erroneously believe that black will result in a more representative sequence than the occurrence of an additional red»,^[10] so people expect that a short run of random outcomes should share properties of a longer run, specifically in that deviations from average should balance out. When people are asked to make up a random-looking sequence of coin tosses, they tend to make sequences where the proportion of heads to tails stays closer to 0.5 in any short segment than would be predicted by chance, a phenomenon known as insensitivity to sample size.^[11] Kahneman and Tversky interpret this to mean that people believe short sequences of random events should be representative of longer ones.^[9] The representativeness heuristic is also cited behind the related phenomenon of the clustering illusion, according to which people see streaks of random events as being non-random when such streaks are actually much more likely to occur in small samples than people expect.^[12]

The gambler’s fallacy can also be attributed to the mistaken belief that gambling, or even chance itself, is a fair process that can correct itself in the event of streaks, known as the just-world hypothesis.^[13] Other researchers believe that belief in the fallacy may be the result of a mistaken belief in an internal locus of control. When a person believes that gambling outcomes are the result of their own skill, they may be more susceptible to the gambler’s fallacy because they reject the idea that chance could overcome skill or talent.^[14]

Variations[edit]

Some researchers believe that it is possible to define two types of gambler’s fallacy: type one and type two. Type one is the classic gambler’s fallacy, where individuals believe that a particular outcome is due after a long streak of another outcome. Type two gambler’s fallacy, as defined by Gideon Keren and Charles Lewis, occurs when a gambler underestimates how many observations are needed to detect a favorable outcome, such as watching a roulette wheel for a length of time and then betting on the numbers that appear most often. For events with a high degree of randomness, detecting a bias that will lead to a favorable outcome takes an impractically large amount of time and is very difficult, if not impossible, to do.^[15] The two types differ in that type one wrongly assumes that gambling conditions are fair and perfect, while type two assumes that the conditions are biased, and that this bias can be detected after a certain amount of time.

Another variety, known as the retrospective gambler’s fallacy, occurs when individuals judge that a seemingly rare event must come from a longer sequence than a more common event does. The belief that an imaginary sequence of die rolls is more than three times as long when a set of three sixes is observed as opposed to when there are only two sixes. This effect can be observed in isolated instances, or even sequentially. Another example would involve hearing that a teenager has unprotected sex and becomes pregnant on a given night, and concluding that she has been engaging in unprotected sex for longer than if we hear she had unprotected sex but did not become pregnant, when the probability of becoming pregnant as a result of each intercourse is independent of the amount of prior intercourse.^[16]

Relationship to hot-hand fallacy[edit]

Another psychological perspective states that gambler’s fallacy can be seen as the counterpart to basketball’s hot-hand fallacy, in which people tend to predict the same outcome as the previous event — known as positive recency — resulting in a belief that a high scorer will continue to score. In the gambler’s fallacy, people predict the opposite outcome of the previous event — negative recency — believing that since the roulette wheel has landed on black on the previous six occasions, it is due to land on red the next. Ayton and Fischer have theorized that people display positive recency for the hot-hand fallacy because the fallacy deals with human performance, and that people do not believe that an inanimate object can become «hot.»^[17] Human performance is not perceived as random, and people are more likely to continue streaks when they believe that the process generating the results is nonrandom.^[18] When a person exhibits the gambler’s fallacy, they are more likely to exhibit the hot-hand fallacy as well, suggesting that one construct is responsible for the two fallacies.^[14]

The difference between the two fallacies is also found in economic decision-making. A study by Huber, Kirchler, and Stockl in 2010 examined how the hot hand and the gambler’s fallacy are exhibited in the financial market. The researchers gave their participants a choice: they could either bet on the outcome of a series of coin tosses, use an expert opinion to sway their decision, or choose a risk-free alternative instead for a smaller financial reward. Participants turned to the expert opinion to make their decision 24% of the time based on their past experience of success, which exemplifies the hot-hand. If the expert was correct, 78% of the participants chose the expert’s opinion again, as opposed to 57% doing so when the expert was wrong. The participants also exhibited the gambler’s fallacy, with their selection of either heads or tails decreasing after noticing a streak of either outcome. This experiment helped bolster Ayton and Fischer’s theory that people put more faith in human performance than they do in seemingly random processes.^[19]

Neurophysiology[edit]

While the representativeness heuristic and other cognitive biases are the most commonly cited cause of the gambler’s fallacy, research suggests that there may also be a neurological component. Functional magnetic resonance imaging has shown that after losing a bet or gamble, known as riskloss, the frontoparietal network of the brain is activated, resulting in more risk-taking behavior. In contrast, there is decreased activity in the amygdala, caudate, and ventral striatum after a riskloss. Activation in the amygdala is negatively correlated with gambler’s fallacy, so that the more activity exhibited in the amygdala, the less likely an individual is to fall prey to the gambler’s fallacy. These results suggest that gambler’s fallacy relies more on the prefrontal cortex, which is responsible for executive, goal-directed processes, and less on the brain areas that control affective decision-making.

The desire to continue gambling or betting is controlled by the striatum, which supports a choice-outcome contingency learning method. The striatum processes the errors in prediction and the behavior changes accordingly. After a win, the positive behavior is reinforced and after a loss, the behavior is conditioned to be avoided. In individuals exhibiting the gambler’s fallacy, this choice-outcome contingency method is impaired, and they continue to make risks after a series of losses.^[20]

Possible solutions[edit]

The gambler’s fallacy is a deep-seated cognitive bias and can be very hard to overcome. Educating individuals about the nature of randomness has not always proven effective in reducing or eliminating any manifestation of the fallacy. Participants in a study by Beach and Swensson in 1967 were shown a shuffled deck of index cards with shapes on them, and were instructed to guess which shape would come next in a sequence. The experimental group of participants was informed about the nature and existence of the gambler’s fallacy, and were explicitly instructed not to rely on run dependency to make their guesses. The control group was not given this information. The response styles of the two groups were similar, indicating that the experimental group still based their choices on the length of the run sequence. This led to the conclusion that instructing individuals about randomness is not sufficient in lessening the gambler’s fallacy.^[21]

An individual’s susceptibility to the gambler’s fallacy may decrease with age. A study by Fischbein and Schnarch in 1997 administered a questionnaire to five groups: students in grades 5, 7, 9, 11, and college students specializing in teaching mathematics. None of the participants had received any prior education regarding probability. The question asked was: «Ronni flipped a coin three times and in all cases heads came up. Ronni intends to flip the coin again. What is the chance of getting heads the fourth time?» The results indicated that as the students got older, the less likely they were to answer with «smaller than the chance of getting tails», which would indicate a negative recency effect. 35% of the 5th graders, 35% of the 7th graders, and 20% of the 9th graders exhibited the negative recency effect. Only 10% of the 11th graders answered this way, and none of the college students did. Fischbein and Schnarch theorized that an individual’s tendency to rely on the representativeness heuristic and other cognitive biases can be overcome with age.^[22]

Another possible solution comes from Roney and Trick, Gestalt psychologists who suggest that the fallacy may be eliminated as a result of grouping. When a future event such as a coin toss is described as part of a sequence, no matter how arbitrarily, a person will automatically consider the event as it relates to the past events, resulting in the gambler’s fallacy. When a person considers every event as independent, the fallacy can be greatly reduced.^[23]

Roney and Trick told participants in their experiment that they were betting on either two blocks of six coin tosses, or on two blocks of seven coin tosses. The fourth, fifth, and sixth tosses all had the same outcome, either three heads or three tails. The seventh toss was grouped with either the end of one block, or the beginning of the next block. Participants exhibited the strongest gambler’s fallacy when the seventh trial was part of the first block, directly after the sequence of three heads or tails. The researchers pointed out that the participants that did not show the gambler’s fallacy showed less confidence in their bets and bet fewer times than the participants who picked with the gambler’s fallacy. When the seventh trial was grouped with the second block, and was perceived as not being part of a streak, the gambler’s fallacy did not occur.

Roney and Trick argued that instead of teaching individuals about the nature of randomness, the fallacy could be avoided by training people to treat each event as if it is a beginning and not a continuation of previous events. They suggested that this would prevent people from gambling when they are losing, in the mistaken hope that their chances of winning are due to increase based on an interaction with previous events.

Users[edit]

Types of users[edit]

Within a real-world setting, numerous studies have uncovered that for various decision makers placed in high stakes scenarios, it is likely they will reflect some degree of strong negative autocorrelation in their judgement.

Asylum judges[edit]

In a study aimed at discovering if the negative autocorrelation that exists with the gambler’s fallacy existed in the decision made by U.S. asylum judges, results showed that after two successive asylum grants, a judge would be 5.5% less likely to approve a third grant.^[24]

Baseball umpires[edit]

In the game of baseball, decisions are made every minute. One particular decision made by umpires which is often subject to scrutiny is the ‘strike zone’ decision. Whenever a batter does not swing, the umpire must decide if the ball was within a fair region for the batter, known as the strike zone. If outside of this zone, the ball does not count towards outing the batter. In a study of over 12,000 games, results showed that umpires are 1.3% less likely to call a strike if the previous two balls were also strikes.^[24]

Loan officers[edit]

In the decision making of loan officers, it can be argued that monetary incentives are a key factor in biased decision making – making it hard to examine the gambler’s fallacy effect. However, research shows that loan officers who are not incentivised by monetary gain are 8% less likely to approve a loan if they approved one for the previous client.^[25]

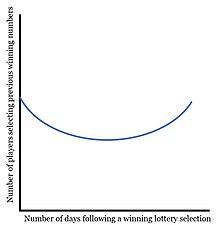
Lottery players[edit]

Lottery play and jackpots entice gamblers around the globe, with the biggest decision for hopeful winners being what numbers to pick. While most people will have their own strategy, evidence shows that after a number is selected as a winner in the current draw, the same number will experience a significant drop in selections in the following lottery. A popular study by Charles Clotfelter and Philip Cook, investigated this effect in 1991, where they concluded bettors would cease to select numbers immediately after they were selected — ultimately recovering selection popularity within three months.^[27] Soon after, a 1994 study was constructed by Dek Terrell to test the findings of Clotfelter and Cook. The key change in Terrell’s study was the examination of a pari-mutuel lottery in which, a number selected with lower total wagers placed on it will result in a higher pay-out. While this examination did conclude that players in both types of lotteries exhibited behaviour in-line with the Gambler’s fallacy theory, those who took part in pari-mutuel betting seemed to be less influenced.^[26]

Table 1. Percentage change in numbers selected by lottery players based on Clotfelter, Cook (1991)^[27]

	Amount bet by lottery players
Numbers drawn 14 April 1988	Draw day	Days after draw
April	Winner Numbers	0	1	3	7	56
11	244	41	34	24	27	30
12	504	29	20	12	18	15
13	718	28	20	17	19	25
14	323	134	95	79	81	76
15	640	10	20	18	16	20
16	957	30	22	20	24	32
Average percentage of players selecting previously winning numbers compared to day of draw	78%	63%	68%	73%

The effect of gambler’s fallacy can be observed as numbers are chosen far less frequently soon after they are selected as winners, recovering slowly over a two-month period. For example, on the 11th of April 1988, 41 players selected 244 as the winning combination. Three days later only 24 individuals selected 244, a 41.5% decrease. This is the gambler’s fallacy in motion, as lottery players believe that the occurrence of a winning combination in previous days will decrease its likelihood of occurring today.

Video game players[edit]

Several video games feature the use of loot boxes, a collection of in-game items awarded on opening with random contents set by rarity metrics, as a monetization scheme. Since around 2018, loot boxes have come under scrutiny from governments and advocates on the basis they are akin to gambling, particularly for games aimed at youth. Some games use a special «pity-timer» mechanism, that if the player has opened several loot boxes in a row without obtaining a high-rarity item, subsequent loot boxes will improve the odds of a higher-rate item drop. This is considered to feed into the gambler’s fallacy since it reinforces the idea that a player will eventually obtain a high-rarity item (a win) after only receiving common items from a string of previous loot boxes.^[28]

See also[edit]

References[edit]

The gambler’s fallacy, also known as the Monte Carlo fallacy or the fallacy of the maturity of chances, is the incorrect belief that, if a particular event occurs more frequently than normal during the past, it is less likely to happen in the future (or vice versa), when it has otherwise been established that the probability of such events does not depend on what has happened in the past. Such events, having the quality of historical independence, are referred to as statistically independent. The fallacy is commonly associated with gambling, where it may be believed, for example, that the next dice roll is more than usually likely to be six because there have recently been fewer than the expected number of sixes.

The term «Monte Carlo fallacy» originates from the best known example of the phenomenon, which occurred in the Monte Carlo Casino in 1913.^[1]

Examples[edit]

Coin toss[edit]

The gambler’s fallacy can be illustrated by considering the repeated toss of a fair coin. The outcomes in different tosses are statistically independent and the probability of getting heads on a single toss is 1/2 (one in two). The probability of getting two heads in two tosses is 1/4 (one in four) and the probability of getting three heads in three tosses is 1/8 (one in eight). In general, if A_i is the event where toss i of a fair coin comes up heads, then:

.

If after tossing four heads in a row, the next coin toss also came up heads, it would complete a run of five successive heads. Since the probability of a run of five successive heads is 1/32 (one in thirty-two), a person might believe that the next flip would be more likely to come up tails rather than heads again. This is incorrect and is an example of the gambler’s fallacy. The event «5 heads in a row» and the event «first 4 heads, then a tails» are equally likely, each having probability 1/32. Since the first four tosses turn up heads, the probability that the next toss is a head is:

.

While a run of five heads has a probability of 1/32 = 0.03125 (a little over 3%), the misunderstanding lies in not realizing that this is the case only before the first coin is tossed. After the first four tosses in this example, the results are no longer unknown, so their probabilities are at that point equal to 1 (100%). The probability of a run of coin tosses of any length continuing for one more toss is always 0.5. The reasoning that a fifth toss is more likely to be tails because the previous four tosses were heads, with a run of luck in the past influencing the odds in the future, forms the basis of the fallacy.

Why the probability is 1/2 for a fair coin[edit]

If a fair coin is flipped 21 times, the probability of 21 heads is 1 in 2,097,152. The probability of flipping a head after having already flipped 20 heads in a row is 1/2. Assuming a fair coin:

• The probability of 20 heads, then 1 tail is 0.5²⁰ × 0.5 = 0.5²¹
• The probability of 20 heads, then 1 head is 0.5²⁰ × 0.5 = 0.5²¹

The probability of getting 20 heads then 1 tail, and the probability of getting 20 heads then another head are both 1 in 2,097,152. When flipping a fair coin 21 times, the outcome is equally likely to be 21 heads as 20 heads and then 1 tail. These two outcomes are equally as likely as any of the other combinations that can be obtained from 21 flips of a coin. All of the 21-flip combinations will have probabilities equal to 0.5²¹, or 1 in 2,097,152. Assuming that a change in the probability will occur as a result of the outcome of prior flips is incorrect because every outcome of a 21-flip sequence is as likely as the other outcomes. In accordance with Bayes’ theorem, the likely outcome of each flip is the probability of the fair coin, which is 1/2.

Other examples[edit]

The fallacy leads to the incorrect notion that previous failures will create an increased probability of success on subsequent attempts. For a fair 16-sided die, the probability of each outcome occurring is 1/16 (6.25%). If a win is defined as rolling a 1, the probability of a 1 occurring at least once in 16 rolls is:

The probability of a loss on the first roll is 15/16 (93.75%). According to the fallacy, the player should have a higher chance of winning after one loss has occurred. The probability of at least one win is now:

By losing one toss, the player’s probability of winning drops by two percentage points. With 5 losses and 11 rolls remaining, the probability of winning drops to around 0.5 (50%). The probability of at least one win does not increase after a series of losses; indeed, the probability of success actually decreases, because there are fewer trials left in which to win. The probability of winning will eventually be equal to the probability of winning a single toss, which is 1/16 (6.25%) and occurs when only one toss is left.

Reverse position[edit]

After a consistent tendency towards tails, a gambler may also decide that tails has become a more likely outcome. This is a rational and Bayesian conclusion, bearing in mind the possibility that the coin may not be fair; it is not a fallacy. Believing the odds to favor tails, the gambler sees no reason to change to heads. However it is a fallacy that a sequence of trials carries a memory of past results which tend to favor or disfavor future outcomes.

The inverse gambler’s fallacy described by Ian Hacking is a situation where a gambler entering a room and seeing a person rolling a double six on a pair of dice may erroneously conclude that the person must have been rolling the dice for quite a while, as they would be unlikely to get a double six on their first attempt.

Retrospective gambler’s fallacy[edit]

Researchers have examined whether a similar bias exists for inferences about unknown past events based upon known subsequent events, calling this the «retrospective gambler’s fallacy».^[2]

An example of a retrospective gambler’s fallacy would be to observe multiple successive «heads» on a coin toss and conclude from this that the previously unknown flip was «tails».^[2] Real world examples of retrospective gambler’s fallacy have been argued to exist in events such as the origin of the Universe. In his book Universes, John Leslie argues that «the presence of vastly many universes very different in their characters might be our best explanation for why at least one universe has a life-permitting character».^[3] Daniel M. Oppenheimer and Benoît Monin argue that «In other words, the ‘best explanation’ for a low-probability event is that it is only one in a multiple of trials, which is the core intuition of the reverse gambler’s fallacy.»^[2] Philosophical arguments are ongoing about whether such arguments are or are not a fallacy, arguing that the occurrence of our universe says nothing about the existence of other universes or trials of universes.^[4][5] Three studies involving Stanford University students tested the existence of a retrospective gamblers’ fallacy. All three studies concluded that people have a gamblers’ fallacy retrospectively as well as to future events.^[2] The authors of all three studies concluded their findings have significant «methodological implications» but may also have «important theoretical implications» that need investigation and research, saying «[a] thorough understanding of such reasoning processes requires that we not only examine how they influence our predictions of the future, but also our perceptions of the past.»^[2]

Childbirth[edit]

In 1796, Pierre-Simon Laplace described in A Philosophical Essay on Probabilities the ways in which men calculated their probability of having sons: «I have seen men, ardently desirous of having a son, who could learn only with anxiety of the births of boys in the month when they expected to become fathers. Imagining that the ratio of these births to those of girls ought to be the same at the end of each month, they judged that the boys already born would render more probable the births next of girls.» The expectant fathers feared that if more sons were born in the surrounding community, then they themselves would be more likely to have a daughter. This essay by Laplace is regarded as one of the earliest descriptions of the fallacy.^[6] Likewise, after having multiple children of the same sex, some parents may erroneously believe that they are due to have a child of the opposite sex.

Monte Carlo Casino[edit]

Perhaps the most famous example of the gambler’s fallacy occurred in a game of roulette at the Monte Carlo Casino on August 18, 1913, when the ball fell in black 26 times in a row. This was an extremely uncommon occurrence: the probability of a sequence of either red or black occurring 26 times in a row is (18/37)^26-1 or around 1 in 66.6 million, assuming the mechanism is unbiased. Gamblers lost millions of francs betting against black, reasoning incorrectly that the streak was causing an imbalance in the randomness of the wheel, and that it had to be followed by a long streak of red.^[1]

Non-examples[edit]

Non-independent events[edit]

The gambler’s fallacy does not apply when the probability of different events is not independent. In such cases, the probability of future events can change based on the outcome of past events, such as the statistical permutation of events. An example is when cards are drawn from a deck without replacement. If an ace is drawn from a deck and not reinserted, the next card drawn is less likely to be an ace and more likely to be of another rank. The probability of drawing another ace, assuming that it was the first card drawn and that there are no jokers, has decreased from 4/52 (7.69%) to 3/51 (5.88%), while the probability for each other rank has increased from 4/52 (7.69%) to 4/51 (7.84%). This effect allows card counting systems to work in games such as blackjack.

Bias[edit]

In most illustrations of the gambler’s fallacy and the reverse gambler’s fallacy, the trial (e.g. flipping a coin) is assumed to be fair. In practice, this assumption may not hold. For example, if a coin is flipped 21 times, the probability of 21 heads with a fair coin is 1 in 2,097,152. Since this probability is so small, if it happens, it may well be that the coin is somehow biased towards landing on heads, or that it is being controlled by hidden magnets, or similar.^[7] In this case, the smart bet is «heads» because Bayesian inference from the empirical evidence — 21 heads in a row — suggests that the coin is likely to be biased toward heads. Bayesian inference can be used to show that when the long-run proportion of different outcomes is unknown but exchangeable (meaning that the random process from which the outcomes are generated may be biased but is equally likely to be biased in any direction) and that previous observations demonstrate the likely direction of the bias, the outcome which has occurred the most in the observed data is the most likely to occur again.^[8]

For example, if the a priori probability of a biased coin is say 1%, and assuming that such a biased coin would come down heads say 60% of the time, then after 21 heads the probability of a biased coin has increased to about 32%.

The opening scene of the play Rosencrantz and Guildenstern Are Dead by Tom Stoppard discusses these issues as one man continually flips heads and the other considers various possible explanations.

Changing probabilities[edit]

If external factors are allowed to change the probability of the events, the gambler’s fallacy may not hold. For example, a change in the game rules might favour one player over the other, improving his or her win percentage. Similarly, an inexperienced player’s success may decrease after opposing teams learn about and play against their weaknesses. This is another example of bias.

Psychology[edit]

Origins[edit]

The gambler’s fallacy arises out of a belief in a law of small numbers, leading to the erroneous belief that small samples must be representative of the larger population. According to the fallacy, streaks must eventually even out in order to be representative.^[9] Amos Tversky and Daniel Kahneman first proposed that the gambler’s fallacy is a cognitive bias produced by a psychological heuristic called the representativeness heuristic, which states that people evaluate the probability of a certain event by assessing how similar it is to events they have experienced before, and how similar the events surrounding those two processes are.^[10][9] According to this view, «after observing a long run of red on the roulette wheel, for example, most people erroneously believe that black will result in a more representative sequence than the occurrence of an additional red»,^[10] so people expect that a short run of random outcomes should share properties of a longer run, specifically in that deviations from average should balance out. When people are asked to make up a random-looking sequence of coin tosses, they tend to make sequences where the proportion of heads to tails stays closer to 0.5 in any short segment than would be predicted by chance, a phenomenon known as insensitivity to sample size.^[11] Kahneman and Tversky interpret this to mean that people believe short sequences of random events should be representative of longer ones.^[9] The representativeness heuristic is also cited behind the related phenomenon of the clustering illusion, according to which people see streaks of random events as being non-random when such streaks are actually much more likely to occur in small samples than people expect.^[12]

The gambler’s fallacy can also be attributed to the mistaken belief that gambling, or even chance itself, is a fair process that can correct itself in the event of streaks, known as the just-world hypothesis.^[13] Other researchers believe that belief in the fallacy may be the result of a mistaken belief in an internal locus of control. When a person believes that gambling outcomes are the result of their own skill, they may be more susceptible to the gambler’s fallacy because they reject the idea that chance could overcome skill or talent.^[14]

Variations[edit]

Some researchers believe that it is possible to define two types of gambler’s fallacy: type one and type two. Type one is the classic gambler’s fallacy, where individuals believe that a particular outcome is due after a long streak of another outcome. Type two gambler’s fallacy, as defined by Gideon Keren and Charles Lewis, occurs when a gambler underestimates how many observations are needed to detect a favorable outcome, such as watching a roulette wheel for a length of time and then betting on the numbers that appear most often. For events with a high degree of randomness, detecting a bias that will lead to a favorable outcome takes an impractically large amount of time and is very difficult, if not impossible, to do.^[15] The two types differ in that type one wrongly assumes that gambling conditions are fair and perfect, while type two assumes that the conditions are biased, and that this bias can be detected after a certain amount of time.

Another variety, known as the retrospective gambler’s fallacy, occurs when individuals judge that a seemingly rare event must come from a longer sequence than a more common event does. The belief that an imaginary sequence of die rolls is more than three times as long when a set of three sixes is observed as opposed to when there are only two sixes. This effect can be observed in isolated instances, or even sequentially. Another example would involve hearing that a teenager has unprotected sex and becomes pregnant on a given night, and concluding that she has been engaging in unprotected sex for longer than if we hear she had unprotected sex but did not become pregnant, when the probability of becoming pregnant as a result of each intercourse is independent of the amount of prior intercourse.^[16]

Relationship to hot-hand fallacy[edit]

Another psychological perspective states that gambler’s fallacy can be seen as the counterpart to basketball’s hot-hand fallacy, in which people tend to predict the same outcome as the previous event — known as positive recency — resulting in a belief that a high scorer will continue to score. In the gambler’s fallacy, people predict the opposite outcome of the previous event — negative recency — believing that since the roulette wheel has landed on black on the previous six occasions, it is due to land on red the next. Ayton and Fischer have theorized that people display positive recency for the hot-hand fallacy because the fallacy deals with human performance, and that people do not believe that an inanimate object can become «hot.»^[17] Human performance is not perceived as random, and people are more likely to continue streaks when they believe that the process generating the results is nonrandom.^[18] When a person exhibits the gambler’s fallacy, they are more likely to exhibit the hot-hand fallacy as well, suggesting that one construct is responsible for the two fallacies.^[14]

The difference between the two fallacies is also found in economic decision-making. A study by Huber, Kirchler, and Stockl in 2010 examined how the hot hand and the gambler’s fallacy are exhibited in the financial market. The researchers gave their participants a choice: they could either bet on the outcome of a series of coin tosses, use an expert opinion to sway their decision, or choose a risk-free alternative instead for a smaller financial reward. Participants turned to the expert opinion to make their decision 24% of the time based on their past experience of success, which exemplifies the hot-hand. If the expert was correct, 78% of the participants chose the expert’s opinion again, as opposed to 57% doing so when the expert was wrong. The participants also exhibited the gambler’s fallacy, with their selection of either heads or tails decreasing after noticing a streak of either outcome. This experiment helped bolster Ayton and Fischer’s theory that people put more faith in human performance than they do in seemingly random processes.^[19]

Neurophysiology[edit]

While the representativeness heuristic and other cognitive biases are the most commonly cited cause of the gambler’s fallacy, research suggests that there may also be a neurological component. Functional magnetic resonance imaging has shown that after losing a bet or gamble, known as riskloss, the frontoparietal network of the brain is activated, resulting in more risk-taking behavior. In contrast, there is decreased activity in the amygdala, caudate, and ventral striatum after a riskloss. Activation in the amygdala is negatively correlated with gambler’s fallacy, so that the more activity exhibited in the amygdala, the less likely an individual is to fall prey to the gambler’s fallacy. These results suggest that gambler’s fallacy relies more on the prefrontal cortex, which is responsible for executive, goal-directed processes, and less on the brain areas that control affective decision-making.

The desire to continue gambling or betting is controlled by the striatum, which supports a choice-outcome contingency learning method. The striatum processes the errors in prediction and the behavior changes accordingly. After a win, the positive behavior is reinforced and after a loss, the behavior is conditioned to be avoided. In individuals exhibiting the gambler’s fallacy, this choice-outcome contingency method is impaired, and they continue to make risks after a series of losses.^[20]

Possible solutions[edit]

The gambler’s fallacy is a deep-seated cognitive bias and can be very hard to overcome. Educating individuals about the nature of randomness has not always proven effective in reducing or eliminating any manifestation of the fallacy. Participants in a study by Beach and Swensson in 1967 were shown a shuffled deck of index cards with shapes on them, and were instructed to guess which shape would come next in a sequence. The experimental group of participants was informed about the nature and existence of the gambler’s fallacy, and were explicitly instructed not to rely on run dependency to make their guesses. The control group was not given this information. The response styles of the two groups were similar, indicating that the experimental group still based their choices on the length of the run sequence. This led to the conclusion that instructing individuals about randomness is not sufficient in lessening the gambler’s fallacy.^[21]

An individual’s susceptibility to the gambler’s fallacy may decrease with age. A study by Fischbein and Schnarch in 1997 administered a questionnaire to five groups: students in grades 5, 7, 9, 11, and college students specializing in teaching mathematics. None of the participants had received any prior education regarding probability. The question asked was: «Ronni flipped a coin three times and in all cases heads came up. Ronni intends to flip the coin again. What is the chance of getting heads the fourth time?» The results indicated that as the students got older, the less likely they were to answer with «smaller than the chance of getting tails», which would indicate a negative recency effect. 35% of the 5th graders, 35% of the 7th graders, and 20% of the 9th graders exhibited the negative recency effect. Only 10% of the 11th graders answered this way, and none of the college students did. Fischbein and Schnarch theorized that an individual’s tendency to rely on the representativeness heuristic and other cognitive biases can be overcome with age.^[22]

Another possible solution comes from Roney and Trick, Gestalt psychologists who suggest that the fallacy may be eliminated as a result of grouping. When a future event such as a coin toss is described as part of a sequence, no matter how arbitrarily, a person will automatically consider the event as it relates to the past events, resulting in the gambler’s fallacy. When a person considers every event as independent, the fallacy can be greatly reduced.^[23]

Roney and Trick told participants in their experiment that they were betting on either two blocks of six coin tosses, or on two blocks of seven coin tosses. The fourth, fifth, and sixth tosses all had the same outcome, either three heads or three tails. The seventh toss was grouped with either the end of one block, or the beginning of the next block. Participants exhibited the strongest gambler’s fallacy when the seventh trial was part of the first block, directly after the sequence of three heads or tails. The researchers pointed out that the participants that did not show the gambler’s fallacy showed less confidence in their bets and bet fewer times than the participants who picked with the gambler’s fallacy. When the seventh trial was grouped with the second block, and was perceived as not being part of a streak, the gambler’s fallacy did not occur.

Roney and Trick argued that instead of teaching individuals about the nature of randomness, the fallacy could be avoided by training people to treat each event as if it is a beginning and not a continuation of previous events. They suggested that this would prevent people from gambling when they are losing, in the mistaken hope that their chances of winning are due to increase based on an interaction with previous events.

Users[edit]

Types of users[edit]

Within a real-world setting, numerous studies have uncovered that for various decision makers placed in high stakes scenarios, it is likely they will reflect some degree of strong negative autocorrelation in their judgement.

Asylum judges[edit]

In a study aimed at discovering if the negative autocorrelation that exists with the gambler’s fallacy existed in the decision made by U.S. asylum judges, results showed that after two successive asylum grants, a judge would be 5.5% less likely to approve a third grant.^[24]

Baseball umpires[edit]

In the game of baseball, decisions are made every minute. One particular decision made by umpires which is often subject to scrutiny is the ‘strike zone’ decision. Whenever a batter does not swing, the umpire must decide if the ball was within a fair region for the batter, known as the strike zone. If outside of this zone, the ball does not count towards outing the batter. In a study of over 12,000 games, results showed that umpires are 1.3% less likely to call a strike if the previous two balls were also strikes.^[24]

Loan officers[edit]

In the decision making of loan officers, it can be argued that monetary incentives are a key factor in biased decision making – making it hard to examine the gambler’s fallacy effect. However, research shows that loan officers who are not incentivised by monetary gain are 8% less likely to approve a loan if they approved one for the previous client.^[25]

Lottery players[edit]

Lottery play and jackpots entice gamblers around the globe, with the biggest decision for hopeful winners being what numbers to pick. While most people will have their own strategy, evidence shows that after a number is selected as a winner in the current draw, the same number will experience a significant drop in selections in the following lottery. A popular study by Charles Clotfelter and Philip Cook, investigated this effect in 1991, where they concluded bettors would cease to select numbers immediately after they were selected — ultimately recovering selection popularity within three months.^[27] Soon after, a 1994 study was constructed by Dek Terrell to test the findings of Clotfelter and Cook. The key change in Terrell’s study was the examination of a pari-mutuel lottery in which, a number selected with lower total wagers placed on it will result in a higher pay-out. While this examination did conclude that players in both types of lotteries exhibited behaviour in-line with the Gambler’s fallacy theory, those who took part in pari-mutuel betting seemed to be less influenced.^[26]

^[27]

	Amount bet by lottery players
Numbers drawn 14 April 1988	Draw day	Days after draw
April	Winner Numbers	0	1	3	7	56
11	244	41	34	24	27	30
12	504	29	20	12	18	15
13	718	28	20	17	19	25
14	323	134	95	79	81	76
15	640	10	20	18	16	20
16	957	30	22	20	24	32
Average percentage of players selecting previously winning numbers compared to day of draw	78%	63%	68%	73%

Table 1. Percentage change in numbers selected by lottery players based on Clotfelter, Cook (1991)

The effect of gambler’s fallacy can be observed as numbers are chosen far less frequently soon after they are selected as winners, recovering slowly over a two-month period. For example, on the 11th of April 1988, 41 players selected 244 as the winning combination. Three days later only 24 individuals selected 244, a 41.5% decrease. This is the gambler’s fallacy in motion, as lottery players believe that the occurrence of a winning combination in previous days will decrease its likelihood of occurring today.

Video game players[edit]

Several video games feature the use of loot boxes, a collection of in-game items awarded on opening with random contents set by rarity metrics, as a monetization scheme. Since around 2018, loot boxes have come under scrutiny from governments and advocates on the basis they are akin to gambling, particularly for games aimed at youth. Some games use a special «pity-timer» mechanism, that if the player has opened several loot boxes in a row without obtaining a high-rarity item, subsequent loot boxes will improve the odds of a higher-rate item drop. This is considered to feed into the gambler’s fallacy since it reinforces the idea that a player will eventually obtain a high-rarity item (a win) after only receiving common items from a string of previous loot boxes.^[28]

See also[edit]

References[edit]

Поделиться статьей:

---

Фальшивая интуиция

Какими бы ни были причины такой фальшивой интуиции, исследования показывают: ошибка игрока может иметь самые серьезные последствия — не только в казино.

Возьмем, к примеру, торговлю на фондовом рынке. Курсы акций часто колеблются в небольших пределах — причем достаточно случайно. Как показал Маттиас Пелстер из Падерборнского университета (Германия), инвесторы могут принимать решения на основе убежденности в том, что цены на акции скоро выровняются. То есть, как и те невезучие итальянцы, они не верят в вероятность колебаний в одну и ту же сторону. И на этом проигрывают.

Ошибка игрока может превратиться в серьезнейшую проблему в тех профессиональных сферах, в которых требуется взвешенное, непредвзятое суждение.

Группа исследователей в США недавно обнаружила, что ложный вывод Монте-Карло влияет на решения судей, предоставляющих убежище беженцам из других стран.

Если рассуждать логически, то порядок рассматриваемых дел не должен иметь никакого значения. Но судьи предоставляли убежище с меньшей вероятностью (до 5,5%), если перед этим уже приняли такое решение в отношении двух соискателей подряд.

Сознательно или нет, они, судя по всему, думали, что три положительных решения подряд — это слишком много.

Затем исследователи проанализировали действия сотрудников банка, рассматривающих заявления о предоставлении кредита. И тут тоже играл роль порядок решений по заявлениям. Отрицательные решения принимались с вероятностью на 8% выше, когда перед этим уже было вынесено два или больше положительных решения. И наоборот.

И наконец, ученые проанализировали действия арбитров матчей Главной лиги бейсбола — и тут тоже обнаружили влияние ложного вывода Монте-Карло на решения спортивных судей. Причем на такие решения, от которых зависел исход матча!

Одна из соавторов исследования, Келли Шу, рассказывает, что ее поразили такие результаты. «Это же профессионалы, принятие таких решений — их главное занятие», — говорит она. И тем не менее…

Более знакомого нам футбола это тоже касается — например, когда в решающем матче дело доходит до серии пенальти. Мячу, чтобы залететь после удара в ворота, требуется 0,2-0,3 секунды.

Голкипер должен очень быстро решить, прыгать ли ему в угол одновременно с ударом или оставаться в центре ворот, надеясь на свою реакцию. По словам Симчи Авугоса из израильского Университета имени Бен-Гуриона, решение вратаря — это фактически азартная игра.

Но, как и работники банка, как и судьи, предоставляющие убежище, вратари чаще всего не верят в то, что все удары подряд могут быть в один и тот же угол.

Коллектив исследователей под руководством Авугоса недавно проанализировал, как пробивались серии пенальти во время финальных матчей Кубка мира и чемпионата Европы. На основе того, что они обнаружили, ученые предлагают футболистам пользоваться тенденцией и продолжать бить в один и тот же угол — ведь вратарь не поверит, что все удары будут в одно место!

И хотя наша повседневная жизнь далека от ситуаций, когда на кон поставлено все, Келли Шу считает, что пресловутая ошибка игрока присутствует практически во всех сферах жизни — даже если мы сами и не осознаем, что прибегаем к подобным вероятностным суждениям.

Шу приводит в пример процесс набора персонала. Если представители работодателя, проводящие собеседование, только что сделали выбор в пользу отличного кандидата, они подсознательно не ожидают, что вслед за ним появится еще один, не менее выдающийся. И этот следующий получит от них более жесткие оценки.

То же самое относится и к учителям, проверяющим сочинения, говорит она. Или, например, вы работник издательства, ищущий новые романы для публикации. Вы можете отказаться от рукописи будущей Джоан Роулинг только на том основании, что уже подписали контракт на пару блестящих рукописей.

Какой бы ни была ваша профессия, вам следует помнить о том хаосе, который породила «лихорадка 53».

Одно и то же событие может происходить много раз подряд, независимо от того, что было до него. И нам стоит призвать на помощь всю свою рациональность и признать: наша интуиция часто подсказывает нам совершенно неверные действия.

На основестатьи Дэвида Робсона. Робсон — автор книги «Ловушка интеллекта: почему умные люди делают глупости».

Даже если вы далеки от азартных игр, все равно можете стать жертвой ситуации выбора под названием ошибка игрока. Чтобы избежать подобных неприятностей, разберемся, что такое ошибка игрока и как ее избежать.

Что такое ошибка игрока?

Ошибка игрока — это когнитивное искажение, выражающееся в неправильном восприятии событий, не связанных между собой. Классический пример ошибки игрока — случай в казино Монте-Карло в начале прошлого века. При игре в рулетку 26 раз подряд выпадало «черное». Игроки ставили на «красное», интуиция подсказывала, что «черная» цепочка должна прерваться, и в очередной раз проигрывали. Ошибку игрока называют еще ложным выводом Монте-Карло.

Чтобы понять, как возникает ошибка игрока, заглянем в теорию вероятностей. Сравните ситуации:

• подбрасывание монеты, в результате которого выпадает орел или решка;

• вытаскивание наугад карты из игральной колоды. Вытащенная карта может быть красной или черной масти.

В обеих ситуациях имеют место два события, но ошибка игрока возникает только в одной из них. В первой — результат очередного броска никак на связан с предыдущими. Вероятность выпадения решки или орла всегда постоянна — ½ . Во второй — вероятность того, что следующей будет вынута карта красной или черной масти равна ½ только при первом вытаскивании, потом вероятность меняется, потому что изменяется соотношение карт разной масти оставшихся в колоде. Чувствуете разницу? Ошибка игрока характерна для событий из разряда первой ситуации.

Ошибка игрока — это попытка уловить закономерность там, где ее нет, как произошло в Монте-Карло. Ошибка игрока связана с фильтрами восприятия и создает ощущение несуществующей связи событий, приводящее к неправильным выводам и действиям. Ошибки игрока встречаются не только в азартных играх, но и в других сферах жизни.

Ошибки игрока, встречающиеся в жизни

Исследования показали, что ошибка игрока влияет на решения судей при вынесении решений о предоставлении убежища беженцам из других стран. Если вынесено два положительных решения подряд, то вероятность того, что третье тоже окажется положительным снижается, хотя события не зависят друг от друга. Аналогичным образом ошибка игрока срабатывает в банках при принятии решений об одобрении кредитов.

Ошибка игрока — ловушка, в которую попадают люди независимо от образования, опыта, стиля мышления. Ошибка игрока может возникать при наборе персонала в компанию. Проведя собеседование с подходящим кандидатом, руководитель подсознательно не ждет, что следующий претендент может быть лучше, оценивает его по более жестким критериям и тем самым занижает важные характеристики, совершая типичную ошибку игрока. Как правильно вести себя на собеседовании, можно прочитать в разделе «Коучинг».

Ошибка игрока часто сбивает с толку людей, проходящих профессиональное тестирование. Если правильный ответ попадает на один и тот же вариант более двух раз подряд, возникают сомнения в собственных знаниях.

Интересен факт — в азартных играх жертвой ошибки становится сам игрок, в жизни ошибка игрока может иметь отрицательные последствия для многих людей. Цена ошибки игрока в реальности может быть очень высокой.

Чтобы не делать ошибок игрока, необходимо уметь отличать события, исход которых не зависит от предыдущих, и не пытаться искать несуществующие закономерности. Помочь может самокоучинг, освоить который можно на программе «Психологическое консультирование и коучинг» в Академии EdPro.

Этот пост написан пользователем Sports.ru, начать писать может каждый болельщик (сделать это можно здесь).

Многие из вас наверняка уже слышали про когнитивные искажения мышления. Признаюсь, что лично я о когнитивной науке узнал сравнительно недавно, хотя сам неосознанно допускал Ошибку игрока (gambler’s fallacy) в бытность моих активных заигрываний с букмекерами.

Обратившись к Википедии, можно узнать, что:

В когнитивной науке под когнитивными искажениями понимаются систематические ошибки в мышлении или шаблонные отклонения, которые возникают на основе дисфункциональных убеждений, внедренных в когнитивные схемы, и легко обнаруживаются при анализе автоматических мыслей. Существование большинства когнитивных искажений было описано учёными, а многие были доказаны в психологических экспериментах.

Когнитивные искажения являются примером эволюционно сложившегося ментального поведения. Некоторые из них выполняют адаптивную функцию, поскольку они способствуют более эффективным действиям или более быстрым решениям. Другие, по-видимому, происходят из отсутствия соответствующих навыков мышления, или из-за неуместного применения навыков, бывших адаптивными в других условиях.

Разработка и применение методов коррекции когнитивных искажений, вызывающих проблемы эмоционального, личностного, социального характера, является предметом различных направлений психотерапии, в частности когнитивной психотерапии.

https://ru.wikipedia.org/wiki/Список_когнитивных_искажений

А вот непосредственно про Ошибку игрока:

Ошибка игрока (англ. gambler’s fallacy) или ложный вывод Монте-Карло отражает распространённое ошибочное понимание случайности событий. Связана с тем, что, как правило, человек не осознаёт на интуитивном уровне того факта, что вероятность желаемого исхода не зависит от предыдущих исходов случайного события.

Например, в случае с подбрасыванием монеты много раз подряд вполне может произойти такая ситуация, что выпадет 9 «решек» подряд. Если монета «нормальная», то для многих людей кажется очевидным, что при следующем броске вероятность выпадения орла будет больше: сложно поверить, что «решка» может выпасть десятый раз подряд. Тем не менее, такой вывод является ошибочным. Вероятность выпадения следующего орла или решки по-прежнему остаётся 1/2.

Нужно, однако, разграничивать понятия: вероятность выпадения «орла» или «решки» в каждом конкретном случае и вероятность выпадения «решки» десять раз подряд. Последняя будет равна {displaystyle (1/2)^{10}=1/1024} (1/2)^{{10}}=1/1024. Впрочем, такой же будет вероятность выпадения и любой другой фиксированной последовательности из «орлов» и «решек» при 10 бросках монеты.

https://ru.wikipedia.org/wiki/Ошибка_игрока

Так в чем же заключалось моё искажение?

Каждый раз, когда английский тур начинался в субботу с первой игры в 15:30 (по Москве), а следующие 6 игр стартовали одновременно в 18:00, я не намеренно, но все-таки где-то в глубине сознания проецировал результат первой вынесенной игры (как сегодня Челси с Арсеналом) на оставшиеся поединки дня. Скажем, если первая игра побивала ТБ, я обретал уверенность, что и в большинстве оставшихся игр тотал будет пройден. Или наоборот, если первая игра была не богатой на голы, то и остальные матчи дня в своем большинстве должны были давать ТМ. Это сейчас я понимаю, насколько несуразными были такие мои выводы Что поделать, игроки в большинстве своем люди суеверные и активно пользуются приметами.

Сейчас я хотел бы спросить моих подписчиков (а их у меня уже 21!), а какие похожие упрощения вы иногда допускаете в ходе анализа предстоящего события? Какие стереотипы могут повлиять на ваши решения? Например:  Лестер ВСЕГДА играет плохо в АПЛ накануне игр в ЛЧ, или если я буду смотреть игру, то моя команда не проиграет (это, кстати, тоже искажение и называется Иллюзией контроля).

Расскажите, пожалуйста, безумно интересно.

Пять дней подряд светило солнце, наверняка завтра будет дождь? Если ваш ответ «да», значит, вы готовы совершить ту же ошибку, что и герой романа Достоевского «Игрок», который советует героине не ставить на «зеро» в рулетку, потому что оно уже выпадало, а дважды за один вечер такого не бывает. Однажды гостям казино Монте-Карло такая уверенность обошлась в целое состояние: чем чаще в рулетке выпадало «черное», тем настойчивее они ставили на «красное», рассчитывая, что теперь-то уж точно выиграют. Но не подозревающий об их расчетах шарик 26 раз подряд останавливался на «черном». 

Что такое ошибка игрока 

Это когнитивное искажение, из-за которого мы видим причинно-следственную связь там, где есть лишь хронологическая последовательность независимых друг от друга событий. Если что-то происходит чаще обычного, нам кажется, что вероятность повторения этого действия с каждым разом уменьшается. И наоборот: если что-то случается реже, чем должно происходить по нашим расчетам, значит, вероятность этого события растет. 

Несмотря на название, это когнитивное искажение действует не только в казино или зале игровых автоматов. Ошибка игрока влияет на многие важные решения, которые мы принимаем на работе и в обыденной жизни. 

Именно в таких реальных условиях это явление изучили исследователи из Цюриха и Бостона. Ученых заинтересовала статистика решений, которые выносят кредитные инспекторы, иммиграционные судьи и бейсбольные рефери. Все они оказались подвержены «негативной автокорректировке». Это означает, что эксперты неосознанно стараются избегать длинных цепочек одинаковых решений. Например, кредитный инспектор, одобрив подряд несколько заявок на кредит, обычно отказывает следующему заявителю, даже если его кредитная история не хуже. Мотив: эксперты знают, что клиенты не могут быть одинаково платежеспособными, поэтому после ряда положительных решений они начинают больше бояться пропустить ненадежного заявителя и придираются к очередной заявке. 

По оценкам исследователей, до 9% отказов в займе могут быть продиктованы ошибкой игрока. На практике это означает, что в 1 из 10 случаев решение о финансировании вашего проекта зависит не от бизнес-плана, а от того, сколько заявок было одобрено перед вашей. Та же закономерность отмечена в судах при рассмотрении заявлений на предоставление статуса беженца и в спорных ситуациях во время игры в бейсбол. Ошибка игрока — универсальное искажение, которому подвержены и специалисты по подбору персонала, и аудиторы, и даже пары, мечтающие о пополнении семьи. Если до этого родились два мальчика, теперь уж точно будет девочка, не так ли? 

Что не так?

Ошибка игрока возникает, как правило, в ситуации выбора, когда есть как минимум две альтернативы. В ее основе — неправильное представление о вероятности. Представьте, что вы подбрасываете монету и у вас пять раз подряд выпадает «решка». Есть ли связь между серией из пяти «решек» и исходом следующего броска? Конечно, нет. Потому что ни один из этих результатов не является ни причиной следующего, ни следствием предыдущего. Это независимые события, которые никак не влияют друг на друга. Доказать это очень просто: допустим, вы не видели результаты пяти предыдущих бросков. Что поменялось? Только ваши ожидания. 

На самом деле вероятность того или иного результата каждый раз остается одинаковой и зависит лишь от того, сколько вариантов у вас есть изначально. В случае с монеткой, у которой всего две стороны, вероятность каждого исхода составляет 1/2. В случае с игровым кубиком вероятность — уже 1/6, потому что у него шесть граней. И сколько бы раз подряд ни выпала шестерка, это не изменит шансы на ее выпадение в следующий раз. 

У многих событий в нашей жизни могут быть сотни, тысячи и даже миллионы вариантов исхода. Например, если вы часто летаете на самолетах, каждый раз риск попасть в авиакатастрофу у вас все равно не больше, чем у того, кто отправляется в свой первый рейс, — 1/1,2 млн. Потому что каждый рейс

— это новый самолет, новые условия, новые пилоты, новый набор факторов и вероятностей. 

Почему мы впадаем в ошибку игрока

Мы не только легко поддаемся этому искажению, иногда оно даже кажется нам проявлением железной логики. Это связано с двумя особенностями психологии восприятия и одним малоизученным нейрофизиологическим механизмом. 

• Первая причина

  — естественная вера в сбалансированность событий. Повторение одного и того же результата кажется нам нарушением вселенского баланса, который обязательно должно исправить следующее событие в серии. Любой перекос требует противовеса. За темной полосой всегда следует светлая. Почему? Потому что это честно. Проблема в том, что монета или игровой кубик за неимением морали и памяти не могут оправдать наши справедливые ожидания, иронизируют Даниэль Канеман и Амос Тверски в книге «Принятие решений в неопределенности: правила и предубеждения». 

• Вторая причина

  — бессознательная склонность объединять ряд разрозненных событий в один процесс просто потому, что они происходят друг за другом. Ученые обнаружили это свойство, изучив реакции компьютерной нейронной сети, которая моделировала образ мышления азартного игрока. Наш мозг воспринимает последовательность однотипных событий как одно событие, разделенное на несколько этапов. И чем меньше временной интервал между ними, тем сильнее это ощущение. Это похоже на эффект мультипликации, в которой иллюзия движения создается за счет быстрой смены не связанных друг с другом картинок. 

Нейробиологические корни ошибки игрока могут быть еще глубже. Проведенное в прошлом году сканирование мозга у 350 испытуемых выявило интересную закономерность: чем меньше объем серого вещества в передней части поясной извилины и медиальной височной доле и чем больше его в полосатом теле и орбитофронтальной коре, тем выше вероятность ошибки игрока. О чем это говорит? Первые две области связаны с когнитивной системой, две другие — с эмоциональной. Авторы исследования считают, что ошибка игрока

— это пример того, как перекос в сторону эмоциональности может нарушать когнитивные функции. 

Эту идею подтверждает и еще одно открытие. Оказывается, ошибке игрока не подвержены люди с повреждением островковой доли. А эта часть мозга тоже участвует в обработке эмоциональной информации. Чтобы «успокоить» гиперактивную островковую долю, ученые рекомендуют медитацию. 

Как не стать ошибающимся игроком 

• Главное

  — научиться отличать взаимосвязанные события от независимых, советует венчурный инвестор и когнитивный исследователь Джефф Стайбел. Если у вас возникает ощущение причинно-следственной связи между двумя событиями, постарайтесь сформулировать для себя хотя бы еще одно логическое объяснение этой связи, помимо того, что события следуют друг за другом. 

• Рассматривайте каждое явление как начало чего-то нового, а не как продолжение последовательности, предлагает автор блога об инвестициях Beginnersbuck финансист Шанкар Нат. 
• Еще один его совет: избавьтесь от иллюзии контроля. Вы не можете предсказывать случайные события, и точка. Мнение, что вы способны на это, мешает принимать решения на основании фактов и анализа данных. 
• И, наконец, повзрослейте: исследования показывают, что с возрастом склонность впадать в ошибку игрока ослабевает. 

Фото на обложке: mohammad alizade / Unsplash

Оши́бка игрока́ (англ. gambler’s fallacy) или ложный вывод Монте-Карло — распространённое ошибочное понимание случайности событий. Связана с тем, что, как правило, человек не осознаёт на интуитивном уровне того факта, что вероятность каждого последующего исхода не зависит от предыдущих исходов случайного события. Однако теория вероятностей рассматривает каждое событие по отдельности как независимое от предыдущих. Несмотря на то, что в первую очередь такое ложное убеждение связывают со сферой азартных игр, оно распространено и в других областях человеческой деятельности и ему подвержены многие люди.

Описание

«Ошибка игрока» представляет собой ошибочное понимание случайности событий, что приводит к убеждению в том, что если в повторяющихся независимых исходах случайного процесса наблюдалось отклонение от ожидаемого поведения, тогда будущие отклонения в противоположном направлении становятся более вероятны. Однако такое умозаключение противоречит теории вероятности, изучающей случайные события, случайные величины. Согласно этой теории необходимо рассматривать каждое событие по отдельности, как статистически независимое от предыдущих, а не в цепи событий. Также в теории вероятности описывается закон больших чисел, формулирующий результат выполнения одного и того же эксперимента много раз. Согласно этому закону, среднее значение конечной выборки из фиксированного распределения близко к математическому ожиданию этого распределения.

В случае с подбрасыванием монеты много раз вполне может произойти такая ситуация, когда выпадет 9 «решек» подряд. Если монета «нормальная» («правильная»), то для многих людей кажется очевидным, что при следующем броске вероятность выпадения «орла» будет больше: сложно поверить, что «решка» может выпасть десятый раз подряд. Тем не менее, такой вывод является ошибочным. Вероятность выпадения следующего орла или решки по-прежнему остаётся 1/2. Эта логика неприменима к случайному вытаскиванию карт из колоды, поскольку количество карт в ней конечно, и чем больше было вытащено, например, черных карт, тем больше вероятность, что следующая будет красная.

Нужно, однако, разграничивать понятия: вероятность выпадения «орла» или «решки» в каждом конкретном случае и вероятность выпадения «решки» [math]displaystyle{ n }[/math] раз подряд (например, два раза подряд или десять раз подряд). Последняя будет равна [math]displaystyle{ (1/2)^{n}= 1/2^{n} }[/math] (для случаев с двумя или десятью выпадениями подряд — соответственно [math]displaystyle{ 1/4 }[/math] или [math]displaystyle{ 1/1024 }[/math]). Впрочем, такой же будет вероятность выпадения и любой другой фиксированной последовательности из «орлов» и «решек» при [math]displaystyle{ n }[/math] бросках монеты.

В целом, если мы представим A_i за событие, то при подбрасывании i правильных монет все они выпадут «орлом» вверх, тогда получается следующий результат:

[math]displaystyle{ Prleft(bigcap_{i=1}^n A_iright)=prod_{i=1}^n Pr(A_i)={1over2^n} }[/math].

Если теперь представить, что мы только что получили четыре последовательных «орла» подряд, так что если пятая монета выпадет «орлом» вверх, то мы закончили цикл из пяти «орлов». Игрок может надеяться, что скорее выпадет «решка» чем «орёл». Однако, это не так, вероятность такого цикла составляет 1/32 (один из тридцати двух). Ошибка заключается в том, что событие выпадения пяти «орлов» подряд равновероятны с событием выпадения четырёх «орлов» и одной «решки», каждое из которых имеет вероятность 1/32. Таким образом при выпадении четырёх «орлов» вероятность выпадения пятого составляет:

[math]displaystyle{ Prleft(A_5|A_1 cap A_2 cap A_3 cap A_4 right)=Prleft(A_5right)=frac{1}{2} }[/math].

Хотя вероятность выпадения пяти «орлов» подряд составляет 1/32 = 0,03125, это вероятность по отношению к первому подбрасыванию. После первых четырёх подбрасываний их исходы уже известны, следовательно их вероятности равняются 1. Утверждение, что вероятность выпадения «решки» в следующем подбрасывании выше из-за предыдущих выпадений «орлов», то есть успехи в прошлом каким-либо образом влияют на шансы в будущем, является заблуждением.

Из предыдущего видно, что, если мы подбросим монету 21 раз, тогда вероятность 21 «орла» составляет 1 из 2 097 152. Однако вероятность получения «орла» после 20 предыдущих «орлов» подряд является 1/2. Такой вариант является применением теоремы Байеса, которая позволяет определить вероятность какого-либо события при условии, что произошло другое статистически взаимозависимое с ним событие.

Рассмотрим такие две вероятности, принимая во внимание, что у нас «правильная» монета:

• вероятность 20 «орлов» и следующей «решки» = 0,5²⁰ × 0,5 = 0,5²¹

• вероятность 20 «орлов» и следующего «орла» = 0,5²⁰ × 0,5 = 0,5²¹

Таким образом обе эти вероятности равняются 1 из 2 097 152. Тогда, равновероятно выбросить 21 «орёл» подряд и 20 «орлов» подряд с последующим одной «решкой». Далее, эти возможности имеют такую ​​же вероятность как и любой другой набор исходов (всего таких 2 097 152); все такие комбинации имеют вероятности равные 0,5²¹ или 1 из 2 097 152. Из этого видно, что нет причин для предположения, что удача изменится в зависимости от предыдущих попыток. Следовательно, как и говорит теорема Байеса, исход каждой попытки сводится к базовой вероятности для «правильной» монеты: ¹⁄₂.

Распространение

Происхождение названия такого когнитивного заблуждения как «ложный вывод Монте-Карло» связывают с событиями, произошедшими 18 августа 1913 года, когда за одним из игровых столов с рулеткой в казино Монте-Карло шарик останавливался на чёрном поле рулетки 26 раз подряд. Как известно, на стандартном колесе рулетки число красных и чёрных ячеек (карманов) одинаковое; следовательно вероятность выпадения одного из цветов равняется чуть меньше 50 % (из-за нуля на рулетке). Однако тогда в Монте-Карло чёрный цвет выпал 26 раз подряд, в связи с чем игроки ставили на красное, надеясь, что последовательность выпадения чёрного прервётся, и проигрывали^[2][3]. Эту историю часто приводят исследователи, занимающиеся психологией азартных игр^[4]. Наблюдения за современными игроками в рулетку показывают, что «ошибка игрока» до сих пор оказывает влияние на выбор, который они делают^[4]. В литературе отмечается, что такой распространённый среди азартных игроков ложный вывод приводит к его использованию в качестве «стратегии Монте-Карло», что является абсолютно неверным умозаключением^[5]. Такое заблуждение иногда ещё называют ошибкой зрелости шансов (англ. fallacy of the maturity of chances)^[6].

Аналогичный хрестоматийный случай имел место в Италии и получил название «лихорадка 53 номера» (итал. la febbre per il 53)^[7][8]. Начиная с 2003 года на протяжении многих розыгрышей итальянской лотереи перестал выпадать выигрышный номер 53. Это совпадение заставило многих людей ставить на это число больше. По наблюдению психолога Дэвида Робсона (англ. David Robson), автора книги «Ловушка интеллекта: почему умные люди делают глупости»^[9], в этом случае имело место также «ошибка игрока»: «…ведь, казалось бы, это очевидно: если число не выпадает так долго, то оно должно выпасть вот-вот!» По его словам, к началу 2005 года «лихорадка 53» привела к банкротству многих людей, некоторые люди кончали жизнь самоубийством, так как упорно ставили на 53-й номер значительные суммы денег и проигрывали: «Массовая истерия завершилась только после того, как 9 февраля число 53 наконец выпало — после того, как не выпадало 182 тиража подряд. За это время на него было поставлено в общей сложности 4 миллиарда евро. Четыре проигранных миллиарда»^[4]. По мнению Робсона: «Какими бы ни были причины такой фальшивой интуиции, исследования показывают: ошибка игрока может иметь самые серьёзные последствия — не только в казино». Такие интуитивные искажения действительности присущи людям не только в сфере азартных игр, но и в других областях человеческой деятельности. Так, зафиксированы случаи применения этой ошибочной стратегии при инвестировании, игре на фондовом рынке^[10][11], в банковской сфере, в судебной практике, при наборе персонала, в спортивных соревнованиях и т. д. Согласно исследованиям отмечается, что люди с более высоким коэффициентом интеллекта предрасположены к этому когнитивному искажению более других, что объясняют тем, что они придают большее значение закономерностям и, таким образом, склонны верить в то, что могут предугадать, какое событие может произойти в следующий раз^[12].

См. также

• Парадокс Берксона
• Задача о разорении игрока

Примечания

Литература

• Каткарт Т., Клейн Д. Как-то раз Платон зашёл в бар…: Понимание философии через шутки. — Альпина диджитал, 2012. — 236 с. — ISBN 978-5-91671-687-0.

Дополнительная литература

• Ayton, P. & Fischer, I. (2004), The hot-hand fallacy and the gambler’s fallacy: Two faces of subjective randomness?, Memory and Cognition Т. 32: 1369–1378, DOI 10.3758/bf03206327
• Barron, Greg & Leider, Stephen (2010), The role of experience in the Gambler’s Fallacy, Journal of Behavioral Decision Making Т. 23 (1): 117–129, ISSN 0894-3257, DOI 10.1002/bdm.676
• Beach, L. R. & Swensson, R. G. (1967), Instructions about randomness and run dependency in two-choice learning, Journal of Experimental Psychology Т. 75: 279–282, DOI 10.1037/h0024979
• Burns, Bruce D. & Corpus, Bryan (2004), Randomness and inductions from streaks: «Gambler’s fallacy» versus «hot hand», Psychonomic Bulletin & Review Т. 11 (1): 179–184, ISSN 1069-9384, DOI 10.3758/BF03206480
• Chen, Daniel; Moskowitz, Tobias J. & Shue, Kelly (2016-03-24), Decision-Making Under the Gambler’s Fallacy: Evidence from Asylum Judges, Loan Officers, and Baseball Umpires*, The Quarterly Journal of Economics: qjw017, ISSN 0033-5533, doi:10.1093/qje/qjw017, <http://qje.oxfordjournals.org/content/early/2016/03/23/qje.qjw017> Архивная копия от 8 августа 2016 на Wayback Machine
• Darling, David. The Universal Book of Mathematics: From Abracadabra to Zeno’s Paradoxes (англ.). — John Wiley & Sons, 2004. — ISBN 978-0-471-27047-8.
• Fischbein, E. & Schnarch, D. (1997), The evolution with age of probabilistic, intuitively based misconceptions, Journal for Research in Mathematics Education Т. 28: 96–105, DOI 10.2307/749665
• Huber, J.; Kirchler, M. & Stockl, T. (2010), The hot hand belief and the gambler’s fallacy in investment decisions under risk, Theory and Decision Т. 68: 445–462, DOI 10.1007/s11238-008-9106-2
• Keren, Gideon & Lewis, Charles (1994), The Two Fallacies of Gamblers: Type I and Type II, Organizational Behavior and Human Decision Processes Т. 60 (1): 75–89, ISSN 0749-5978, DOI 10.1006/obhd.1994.1075
• Lehrer, Jonah  (англ.) (рус.. How We Decide (неопр.). — New York: Houghton Mifflin Harcourt  (англ.) (рус., 2009. — ISBN 978-0-618-62011-1.
• O’Neill, B. & Puza, B.D. (2005), In defence of the reverse gambler’s belief, The Mathematical Scientist Т. 30 (1): 13–16, ISSN 0312-3685
• Oppenheimer, D. M. & Monin, B. (2009), The retrospective gambler’s fallacy: Unlikely events, constructing the past, and multiple universes, Judgment and Decision Making Т. 4: 326–334
• Rogers, Paul (1998), The cognitive psychology of lottery gambling: A theoretical review, Journal of Gambling Studies Т. 14 (2): 111–134, ISSN 1050-5350, DOI 10.1023/A:1023042708217
• Roney, C. J. & Trick, L. M. (2003), Grouping and gambling: A gestalt approach to understanding the gambler’s fallacy, Canadian Journal of Experimental Psychology Т. 57: 69–75, DOI 10.1037/h0087414
• Suetens, Sigrid; Galbo-Jørgensen, Claus B. & Tyran, Jean-Robert (2016-06-01), Predicting Lotto Numbers: A Natural Experiment on the Gambler’s Fallacy and the Hot-Hand Fallacy, Journal of the European Economic Association Т. 14 (3): 584–607, ISSN 1542-4774, doi:10.1111/jeea.12147, <http://onlinelibrary.wiley.com/doi/10.1111/jeea.12147/abstract> Архивная копия от 28 января 2018 на Wayback Machine
• Sundali, J. & Croson, R. (2006), Biases in casino betting: The hot hand and the gambler’s fallacy, Judgment and Decision Making Т. 1: 1–12
• Gilovich, Thomas (1991), How we know what isn’t so, New York: The Free Press, с. 16–19, ISBN 0-02-911706-2
• Tune, G. S. (1964), Response preferences: A review of some relevant literature, Psychological Bulletin Т. 61 (4): 286–302, PMID 14140335, DOI 10.1037/h0048618
• Tversky, Amos & Daniel Kahneman (1971), Belief in the law of small numbers, Psychological Bulletin Т. 76 (2): 105–110, DOI 10.1037/h0031322
• Tversky, Amos & Daniel Kahneman (1974), Judgment under uncertainty: Heuristics and biases, Science Т. 185 (4157): 1124–1131, PMID 17835457, DOI 10.1126/science.185.4157.1124
• Xue, G.; Lu, Z.; Levin, I. P. & Bechara, A. (2011), An fMRI study of risk-taking following wins and losses: Implications for the gambler’s fallacy, Human Brain Mapping Т. 32: 271–281, DOI 10.1002/hbm.21015

Ссылки

• Ошибка игрока Архивная копия от 4 сентября 2019 на Wayback Machine (англ.) на сайте Easy Vegas Архивная копия от 29 февраля 2020 на Wayback Machine
• Ошибка игрока Архивная копия от 29 февраля 2020 на Wayback Machine (англ.) на сайте PokerMoments Архивная копия от 29 февраля 2020 на Wayback Machine